Mysteries of Odd Mathematics
Math is the Language of All Sciences
Anything that has a pattern has a mathematical explanation to it. Some things I have yet to figure out, other things I've figured out a while back.
When I was in elementary school, someone told me that if you add all the digits of a number together (for the number 12, add 1 and 2 to get 3) if the number was divisible by 3 the sum would be 3, 6, or 9. They went on to state the reverse that if the sum is either 3, 6, or 9 that number would be divisible by 3. It doesn't matter if there are ten digits to add together or just two, the rule has never proven wrong for me.
And I was curious to see if there were any more patterns. I wanted to solve for them as opposed to just look them up. Most that I did eventually solve I found had been solved for years earlier in other places and by less pretty people than myself. For example, I discovered that if you add the digits of any number together and they sum up to 9, that number is divisible by 9. That was my first one and I since have seen it in many number theory sources. Oh well.
But there are others. Many, many others. For example... my first "big" solve. I was playing with exponents. I listed off all the numbers that were squares. 1, 4, 9, 16, 25, 36, 49... so on and so on. The differences between the numbers gets bigger. Between 1 and 4 the difference is only 3 where between 36 and 49 it is 13. I discovered that the differences between the squares was sequential, but I didn't have the mathematical background to describe it well. It hides in plane sight that the differences grow two at a time (from 3 to 5 to 7 to 9 to 11 to 13). Pretty sweet, eh? So if you ever want to predict what the next square in the sequence is, simply find the difference between the last two known squares, add two to that difference, then add that to the last number in the sequence. See for yourself in the following example: I want to know what 8 squared is going to be. I find 7 squared and subtract 6 squared (49-36 =13) I add 2 to 13, which is 15. I then add 15 to 49 to get 64, which is 8 squared.
And that was the beginning of a bigger adventure. This shit never gets old to me.
The First Leg of the Grand Adventure
Studying squares quickly led to a dead-end, except for if you looked at cubes as well. For those of you who don't know, a square in math terms is not a shape, it's when you multiply a number by itself once, say 8*8=64. A cube is when you multiply a number by itself twice, say 8*8*8=512. Beyond that, they say eight raised to the fourth power for 8*8*8*8 = 4,096 or raised to the fifth power. Every once in a while you'll hear someone refer to it as eight raised to the seventh exponent. Same thing.
Anyway, I quickly got bored listing off as many numbers squared as I could to see if the rule held true. It is, just take my word for it now. But cubes? At first test, I found that the differences were junk. At second glance, I realized not so much. You had to take the differences between the differences to solve the pattern. There is no way I can explain it in words, so let me explain the image below. The first line is a row of first few numbers cubed. They are highlighted red. The differences between the numbers in the first row are listed below and in between their respective numbers and are highlighted in green. The difference in between the differences are below those and are highlighted in blue.
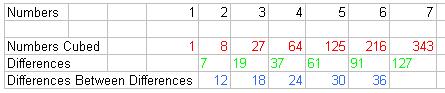
Not the Obvious
So you can see that the green numbers don't look like they have a pattern, but they do. The blue numbers are all 6 apart from each other. If I were to want to predict the next blue, it's easy: 36+6=42. If I want to predict the next green, it's easy: 127+42=169. If I want to predict the next red, it's still pretty easy: 169+343=512. Sure enough, 8 cubed is 512.
There was something I hadn't discovered until I got at least this far. The larger exponent you're working with, the more differences between differences you'll need. When working with an exponent of 2, you only need 2 layers: the red and the green. When working with an exponent of 3, you need 3 layers: red, green, and blue. I went on to study exponents of 4, 5, and 6 before I figured out that yes, for whatever exponent you are using, you need that many layers. To predict the next exponent in the sequence, you need the same amount of numbers already solved. For example, if you are using exponents of two, you'll need to take the difference between two numbers. If you're using exponents of three, you'll need to take the differences between three numbers. If you are using exponents of four, you'll need to take differences between four numbers. So on and so on.
And that ain't the end of it, sister. Notice how the differences between the blues is sequential, but has 6 in between each one? When working with squares, the differences were also sequential but were only 2 apart? The number you will need when you reach your last "layer" is what mathematicians call the factorial of your exponent. This means that you multiply a number by all the numbers lower than it and is designated by the exclamation point. 4 factorial would be represented as 4! = 4*3*2*1 = 24. So when you're trying to solve for the next number raised to the fourth power, you need to go to the fourth layer and add 4!. Score!
Another very useless way to write an equation to solve for an exponent is say 8^4 = (#^4)^(Log(base#)8). The reason it's useless is because you'll spend five times as much effort to solve it with this equation as you would just crunching out the numbers.