The Twin Paradox
The twin paradox is an example used in relativity. In the story there are twin brothers. One of the brothers takes a long trip, in a spacecraft, moving at a speed close the speed of light, both going and returning, while the other brother stays home. The speed of the brother taking the trip causes his time to move slower than his stay at home brother. Hence he will not age as fast as his brother at home. Therefore when he returns he finds that his twin brother is now older than him. If we view the event from the reference frame of the brother on the spacecraft, he can perceive that his stay at home twin is moving away at high speed relative to his spacecraft. Thus time will move slower for the stay a home twin, who will now be younger than his twin when they are reunited. This presents the paradox, each twin thinks that when they reunite, his brother will be older than him.
Here we will use a modified x,t Minkowski space-time diagram and a twin pair of docs (fig. 1) to illustrate the twin paradox. In this 2-D diagram the vertical time axis is measured in years. The horizontal spatial axis represents distance in light years, that is, the distance that light would travel through space in one year relative to the observers frame. Thus the time and space units of the reference frame are drawn to the same length. There are several time axes each with their own scale, one for each different velocity relative to the reference frame. These are in years as measured by observers traveling at different velocities. Since the scales are partly obscured by the twins, the scales are also duplicated at the sides of the diagram.
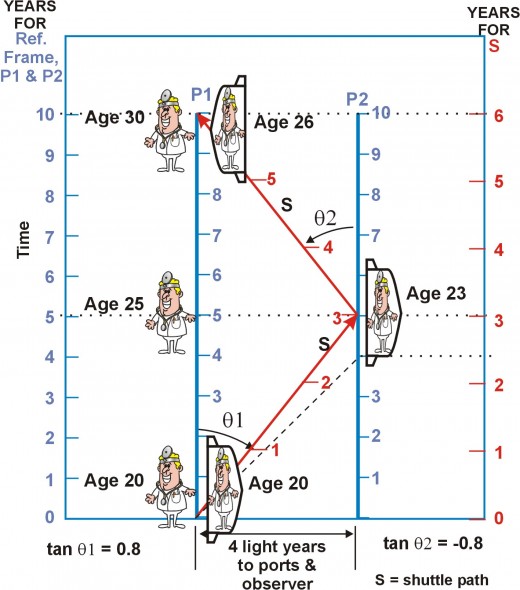
In fig. 2 there are two space ports, 4 light years apart. Both are stationary to each other and to us the external observer. On space port one (P1), there are twin brothers who are 20 years old. One brother will fly a space shuttle S from P1 to P2 to deliver supplies and then return to P1. He leaves in the year 2100 and travels the distance to P2 at an average velocity of 0.8c. At this velocity it will take him 5 years to reach P2. Because of his velocity relative to the observer and the space ports, his time will progress at a rate tr = 1/(1-[v/c]2)1/2 = 5/3 slower than time on either space port. When he arrives at P2 he has aged only 3 years, but it is the year 2105 at P2. After making his delivery he returns to P1 with an average velocity of -0.8c. This will require 3 years of his time but another 5 years have passed on the space port. When he arrives at P1 he is now 26 years old, but it is 2110 on P1 and his twin brother is 30 years old. On the diagram the slope of the velocity of light (c) is represented as a dashed line and at a 45 degree angle to either axis since the arctan c/c = 45 degrees.
One could assume that the space ports are moving and the brother in the shuttle is stationary. That would mean that the shuttle rider ages 10 years while the stay-at-homes only ages 6 years. That assumption creates the twin paradox. Let us look at two other views of this same event.
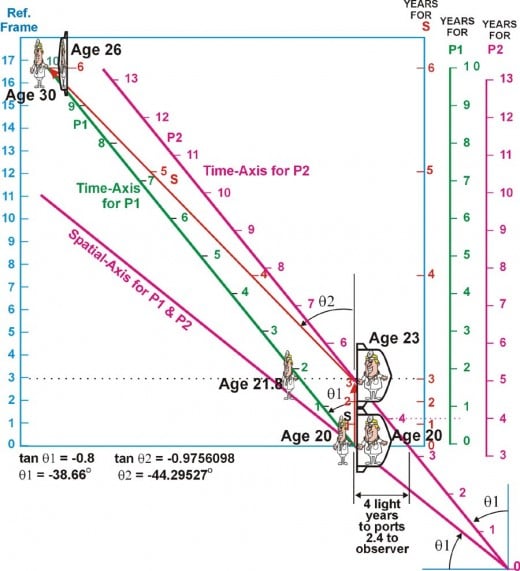
What if the two space ports were still stationary to each other and observers on the ports measure their distance as 4 light years apart? However, in fig. 3, both ports are moving relative to an independent observer at a velocity of -0.8c. This would mean that to the independent observer they are only 2.4 light years apart, since distance has contracted for objects moving relative to the observer.
When the brother in the shuttle accelerates to 0.8c relative to P1 he has now become stationary to the observer. To the observer, he is now aging faster, rather than slower than his brother. It will still take 3 of the traveler's years for P2 to arrive at his position in space. He will decelerate to dock with P2, by accelerating to -0.8c. When he docks on P2 his age is 23 while his brother back on P1 is only 21.8 years old. But the year on P2 is 2105 because of synchronization of the clocks between P1 and P2. To return to P1 with a relative speed of 0.8 to P1 and P2, he must return at a velocity of (using the addition of velocity equation)
vA+B = (vA+vB)/(1+vAvB)/c2) = (-0.8c+-0.8c)/(1+(-0.8c x -0.8c)/c2)
= -1.6c/1.64 = -0.9756098c
relative to the observer. Thus his time rate
Tr = TO/[1-(v/c)2]1/2 = 1/0.219512 = 4.555596
This will cause his time to advance 4.5555 times slower than when he was stationary relative to the observer. The time it would take for the shuttle to catch up to P1 is ΔT = distance/overtake velocity = 2.4 light years/(0.9756-0.8) = 13.6667 years. Therefore he will age only (age = ΔT/Tr) 13.6667/4.5555 = 3.0 years on his return trip. To the observer this total trip took 3 years + 13.6667 years = 16.6667 years.
Since the stay at home brother has a relative velocity to the observer of 0.8c his time rate is
Tr = TO/[1-(v/c)2]1/2 = 1/0.6 = 1.6667.
Thus this brother will age 16.6667/1.6667 = 10 years during his brother's trip. When the traveler returns he is 20+3+3 = 26 and the stay-at-home brother is 20+10 = 30. This is the same as before. It is the twin that is accelerated to a very high speed, in order to catch up to his brother that is younger when the brothers are reunited.
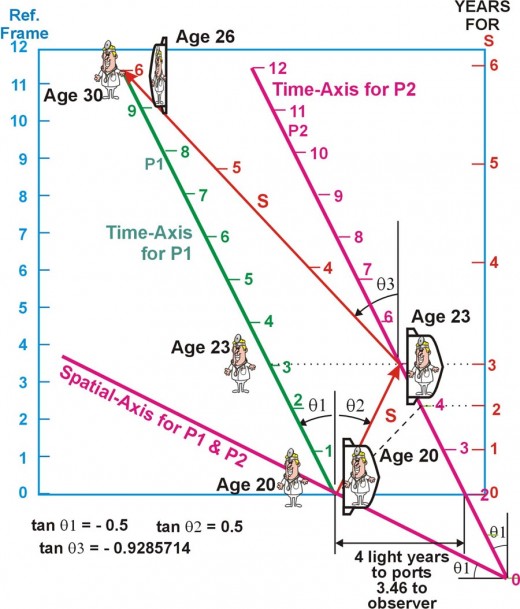
We have just seen that it made no difference in the end results whether the space ports were stationary to or moving at -0.8c relative to the observer. What if they were moving at some other speed, as in fig. 4? If the ports were moving at -0.5c relative to the observer, what velocity would the shuttle have to obtain in order to have a relative velocity of 0.8c to the ports? Let's call the relative velocity 0.8c, rv, the port velocity -0.5c, pv, and the shuttle velocity sv.
sv = (rv + pv)/(1+(rv x pv)/c2) = (0.8c + - 0.5c)/(1+(0.8c x -0.5c)/c2) = 0.3c/0.6 = 0.5c.
Since both brothers are moving at the same velocity, they both age the same while the shuttle is on its way to P2. Here again it takes 3 of the traveler's years for the shuttle to reach P2 and the year it arrives is again 2105. It will take 3 x 1/(1-.52)1/2 = 3/0.866 = 3.4641 years in the observer's time. In order to return to P1 at a relative velocity of 0.8c he must travel at a velocity of (using the velocity addition equation)
sv = (-0.8c + -0.5c)/(1+(-0.8c x -0.5c)/c2) = -1.3c/1.4 = -0.92857143c
relative to the observer. Thus his time rate
Tr = TO/[1-(v/c)2]1/2 = 1/0.37115374 = 2.694301282
This will cause his time to advance 2.6943013 times slower than when he was stationary relative to the observer. The time it would take for the shuttle to catch up to P1 is ΔT = 3.4641 light years/(0.92857143-0.5) = 8.0829269 years. Therefore he will age only (age = ΔT/Tr) 8.0829269/2.6943013 = 3.0 years on his return trip. To the observer this total trip took 3.4641 years + 8.0829269 years = 11.547 years.
Since the stay at home brother has a relative velocity to the observer of 0.5c his time rate is
Tr = TO/[1-(v/c)2]1/2 = 1/0.86667 = 1.1547.
Thus this brother will age 11.547/1.1547 = 10 years. When the traveler returns he is 26 and the stay-at-home brother is 30.
From these 3 cases, we see it doesn't matter from which viewpoint the event is observed; it is their relative velocity to each other that determines their age difference. And it is the brother who accelerates to reunite with his brother that ages the least. Hence the twin paradox is not a paradox at all.