Advanced Proofs of the Dot and Cross Products for Calculus

Vectors have so many properties that make their versatility endless and crossover into several disciplines. Sometimes we add them or subtract them, sometimes we take the dot product to find a scalar or a cross product to find a perpendicular vector to two other ones, but other times we may be interested in finding a vector that bisects two vectors. How could we make such a vector? Below we will show how to find that vector which bisects a and b, which happens to be c = ||b||a + ||a||b (Larson 772).

To find such a vector (let’s call it c), I need to remember that it will be the same number of degrees from a as it will be from b. Using one of the definitions for the dot product, I know that

Cos Θ = (c ∙ b)/(||c|| ||b||)

And

Cos Θ = (c ∙ a)/(||c|| ||a||)

But since this is the same number of degrees, both cosine terms will equal each other. Therefore

(c ∙ b)/(||c|| ||b||) = (c ∙ a)/(||c|| ||a||)

Or after eliminating the common term ||c||

(c ∙ b)/(||b||) = (c ∙ a)/(||a||)

Multiplying both sides by ||a|| ||b|| gives us

||a||(c ∙ b) = ||b||(c ∙ a)

And upon expanding the dot product we arrive at

||a||(c₁b₁ + c₂b₂ + c₃b_{3) =} ||b|| (c₁a₁ + c₂a₂ + c₃a₃)

Distributing the outside term results in

||a||c₁b₁ + ||a||c₂b₂ + ||a||c₃b_{3 =} ||b||c₁a₁ + ||b||c₂a₂ + ||b||c₃a₃

And after moving all the terms to the left-hand side we have

||a||c₁b₁ -||b||c₁a₁ + ||a||c₂b₂ - ||b||c₂a₂ + ||a||c₃b₃ - ||b||c₃a₃ = 0

Pulling out common c terms will give us

c₁(||a||b₁ -||b||a₁) + c₂(||a||b₂ - ||b||a₂) + c₃(||a||b₃ - ||b||a₃) = 0

Now, some may remember the definition of the conjugate. If I have a (x + y) term, if I multiply it by (x - y), I get x² + xy – xy – y² = x² – y². Simply put, I multiply by the same term but I change the operation being done to the opposite partner, often in hopes of simplifying further algebra. Therefore, if the components of c are conjugates, then

c₁ = ||a||b₁ + ||b||a_1, c₂ = ||a||b₂ + ||b||a_2, and c₃ = ||a||b₃ + ||b||a₃

Plugging these back in to our equation gives us

(||a||b₁ + ||b||a₁ )(||a||b₁ -||b||a₁) + (||a||b₂ + ||b||a₂)(||a||b₂ - ||b||a₂) + (||a||b₃ + ||b||a₃)(||a||b₃ - ||b||a₃)

= ||a||²b₁² -||a||b₁||b||a₁ + ||a||b₁||b||a₁ -||b||²a₁² + …

= ||a||²b₁² - ||b||²a₁² + ||a||²b₂² - ||b||²a₂² + ||a||²b₃² - ||b||²a₃²

And after pulling out some common terms we arrive at

=||a||² (b₁² + b₂² + b₃²) - ||b||² (a₁² + a₂² + a₃²)

But b₁² + b₂² + b₃² is the length squared, or ||b||², and the same applies for the a terms, so

||a||² (b₁² + b₂² + b₃²) - ||b||² (a₁² + a₂² + a₃²) = ||a||² ||b||² - ||b||² ||a||² = 0

Yes, c is the conjugate, so

c = <c₁, c₂, c₃> = <||a||b₁ + ||b||a_1, ||a||b₂ + ||b||a_2, ||a||b₃ + ||b||a₃> = ||b||a + ||a||b

When I take the difference of two vectors, I will have a net vector as my result. It too will have a magnitude to it, but how is it possible for me to relate this length to the dot product? Below we will show that ||a- b||² = ||a||² + ||b||² – 2(a ∙ b) is true (789).

First, note that by definition of vector magnitude,

||a- b||² = ([(a₁ – b₁)² + (a­₂ – b­₂)² + (a₃ – b­₃)²]^0.5)²

=(a₁ – b₁)² + (a­₂ – b­₂)² + (a₃ – b­₃)²

Expanding this out gives us

(a₁ – b₁)(a₁ – b₁) + (a­₂ – b­₂)(a­₂ – b­₂) + (a₃ – b­₃)(a₃ – b­₃)

= a₁² – a­₁b_­1 – a­₁b₁ + b­₁² + a₂² – a­₂b_­2 – a­₂b₂ + b­₂² + a₃² – a­₃b_­3 – a­₃b₃ + b­₃²

= a₁² – 2a­₁b_­1 + b­₁² + a₂² – 2a­₂b_­2 + b­₂² + a₃² – 2a­₃b_­3 + b­₃²

And after a little bit of rearrangement, we will have this equaling

a₁² + a₂² + a₃² + b­₁² + b­₂² + b­₃² – 2a­₁b_­1 – 2a­₂b_­2 – 2a­₃b_­3

But notice that

a₁² + a₂² + a₃² = [(a₁² + a₂² + a₃²)^0.5]² = ||a||²

b₁² + b₂² + b₃² = [(b₁² + b₂² + b₃²)^0.5]² = ||b||²

– 2a­₁b_­1 – 2a­₂b_­2 – 2a­₃b_­3 = -2(a­₁b_­1 + a­₂b_­2 + a­₃b_­3) = -2 (a ∙ b)

So

a₁² + a₂² + a₃² + b­₁² + b­₂² + b­₃² – 2a­₁b_­1 – 2a­₂b_­2 – 2a­₃b_­3 = ||a||² + ||b||² – 2(a ∙ b).

Though often used in linear algebra, this inequality is dependent on the definition of the dot product if it is to work at all. It states that |a∙ b| ≤ ||a|| ||b||, or that the absolute value of the dot product is less than or equal to the product of the magnitudes of a and b (789). Here is how we know this to be true.

First, we need to use one of the definitions of the dot product.

|a∙ b| = | ||a|| ||b|| Cos Θ |

Now we need to take a look at the possible values for Θ. When Θ = 0, Cos 0 = 1 so

|a∙ b| = | ||a|| ||b|| Cos 0 | = ||a|| ||b||

When Θ = 90, Cos 90 = 0 so

|a∙ b| = | ||a|| ||b|| Cos 90 | = 0.

Now, for the range 0 ≤ Θ ≤ 90, our cosine term is between zero and one, so the absolute value of the dot product is between zero and ||a|| ||b||. But what about the range of 90 ≤ Θ ≤ 180? Our cosine terms would be between zero and negative one, but because this is the absolute value of both sides, the net value is still the same as it would be for the 0 ≤ Θ ≤ 90 range. So from 0 to π the greatest |a∙ b| can be is

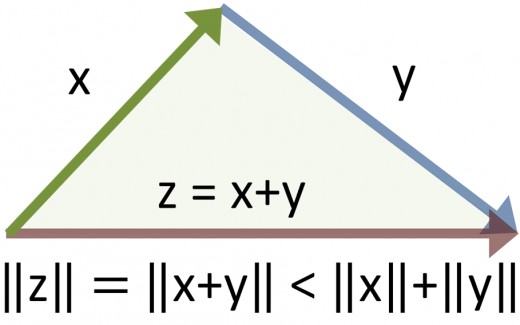
As the name implies, this identity stems from a triangle, specifically from the Law of Cosines. The actually inequality is ||a + b|| ≤ ||a|| + ||b||, or that the magnitude of the vector sum a + b is less than or equal to the actual sum of the magnitudes of a and b (789). Now, for the proof. The Law of Cosines states that

c² = a² + b² – 2abCosΘ

Or that the long side of a triangle squared will equal the squares of both sides minus two times the sides and cosine of the angle opposite the long side of the triangle. If we make a triangle with short sides of ||a|| and ||b|| with a long side of ||a + b|| then we will have

||a + b||² = ||a||² + ||b||²- 2 ||a|| ||b|| CosΘ

Now, we will have several cases to examine. First, if Θ = 0, then Cos0 = 1 so

||a + b||² = ||a||² + ||b||²- 2 ||a|| ||b|| = (||a|| - ||b||)²

Or, after taking the square root of both sides,

||a + b|| = ||a|| - ||b||

Which is definitely less than ||a|| + ||b||, as the inequality states.

Now, what if Θ = 90? Then Cos90 = 0 so

||a + b||² = ||a||² + ||b||²

Which is not only the Pythagorean Theorem but it is also less than ||a||² + ||b||² + 2 ||a|| ||b||, or

||a + b||² < ||a||² + ||b||² + 2 ||a|| ||b|| = (||a|| + ||b||)²

And after taking the square root of both sides, we have

||a + b|| < ||a|| + ||b||.

Just as we expect if the Triangle Inequality is true.

If Θ = 180 (which it couldn’t if this were a triangle), Cos180 = -1 so

||a + b||² = ||a||² + ||b||²- 2 ||a|| ||b|| (-1) = ||a||² + ||b||²+ 2 ||a|| ||b|| = (||a|| + ||b||)²

Or after taking the square root of both sides

||a + b|| = ||a|| + ||b||

Again, a result that the Triangle Inequality states is possible. It is interesting to point out what this would look like. In this case, a and b would both be pointing in opposite directions, so the length of a + b would have to be the lengths of a and b individually.

So are we done with the proof? Not quite. What about for the theta range 0 ≤ Θ ≤ 90? That means that 1 ≤ CosΘ ≤ 0 and that everything on the right hand of the Law of Cosines is going to be greater than ||a|| - ||b|| but less than ||a||² + ||b||², which we know from both cases will be bigger than ||a + b||. We can use a similar argument for the range 90 ≤ Θ ≤ 180.

As the title implies, we will explore what the cross-cross product is. Below is the proof that will show that a x (b x c) = (a ∙ c)b - (a ∙ b)c (797). Note that

a x (b x c) = a x [i(b­₂c₃ – b­_­3­­c₂) - j(b₁c₃ – b₃c₁) + k(b₁c₂ – b₂c₁)]

Which, if I write it in determinant form is

| i j k |

= | a₁ a₂ a₃ |

| b­₂c₃ – b­_­3­­c₂ -b₁c₃ + b₃c₁ b₁c₂ – b₂c₁ |

And that can be rewritten after taking the determinant as

i[a₂(b₁c₂ – b₂c₁) – a₃(-b₁c₃ + b₃c₁)] – j[a₁(b₁c₂ – b₂c₁) – a₃(b­₂c₃ – b­_­3­­c₂)] + z[a₁(-b₁c₃ + b₃c₁) - a₂(b₂c₃ – b­_­3­­c₂)]

Oh boy, ready for this algebra?

i[a₂b₁c₂ – a₂b₂c₁ + a₃b₁c₃ –a­₃b₃c₁] – j[a₁b₁c₂ – a­₁b₂c₁ – a₃b­₂c₃ + a₃b­_­3­­c₂] + z[-a₁b₁c₃ + a₁b₃c₁ - a₂b₂c₃ + a₂b­_­3­­c₂]

= i[a₂b₁c₂ + a₃b₁c₃ – a₂b₂c₁–a­₃b₃c₁] – j[– a­₁b₂c₁ – a₃b­₂c₃ + a₃b­_­3­­c_{2 +} a₁b₁c₂] + z[a₁b₃c₁ + a₂b­_­3­­c₂ - a₁b₁c₃ - a₂b₂c₃]

And after distributing negative signs and distributing unit vectors we have

i[a₂b₁c₂ + a₃b₁c₃] –i[ a₂b₂c₁ + a­₃b₃c₁] + j[a­₁b₂c₁ + a₃b­₂c₃] - j[a₃b­_­3­­c_{2 +} a₁b₁c₂] + z[a₁b₃c₁ + a₂b­_­3­­c₂] – z[a₁b₁c₃ + a₂b₂c₃]

And If I pull out select b and c terms we will have

ib₁[a₂c₂ + a₃c₃] –ic₁[a₂b₂+ a­₃b₃] + jb₂[a­₁c₁ + a₃c₃] - jc₂[a₃b­_{­3­­ +} a₁b₁] + zb₃[a₁c₁ + a₂c₂] – zc₃[a₁b₁ + a₂b₂]

= ib₁[a₂c₂ + a₃c₃] + jb₂[a­₁c₁ + a₃c₃] + zb₃[a₁c₁ + a₂c₂] –ic₁[a₂b₂+ a­₃b₃] - jc₂[a₃b­_{­3­­ +} a₁b₁]– zc₃[a₁b₁ + a₂b₂]

Now, if I add and subtract specific ab and ac terms so the sum of them is zero, I will have

ib₁[a₁c₁+ a₂c₂ + a₃c₃ - a₁c₁] + jb₂[a­₁c₁ + a₂c₂ + a₃c₃ - a₂c₂] + zb₃[a₁c₁ + a₂c₂ + a₃c₃ - a₃c₃] –ic₁[a₁b₁ + a₂b₂ + a­₃b₃ - a₁b₁] - jc₂[a₁b₁ + a₂b₂ + a₃b­_­3­­ - a₂b₂]– zc₃[a₁b₁ + a₂b₂ + a₃b­_­3­­ - a₃b­_­3­­]

But notice now that we have dot products! Further simplifying this now is

ib₁[a ∙ c- a₁c₁] + jb₂[a ∙ c- a₂c₂] + zb₃[a ∙ c- a₃c₃] – ic₁[a ∙ b - a₁b₁] - jc₂[a ∙ b - a₂b₂]– zc₃[a ∙ b - a₃b­_­3­­]

And after further distributing the terms, we have

ib₁[a ∙ c]- ib₁a₁c₁ + jb₂[a ∙ c]- jb₂a₂c₂ + zb₃[a ∙ c]- zb₃a₃c₃ – ic₁[a ∙ b] + ic₁a₁b₁ - jc₂[a ∙ b] + jc₂a₂b₂ – zc₃[a ∙ b] + zc₃a₃b­_­3­­

Notice how we have abc terms cancel out, so after eliminating those we have

ib₁[a ∙ c] + jb₂[a ∙ c]+ zb₃[a ∙ c] – ic₁[a ∙ b] - jc₂[a ∙ b] – zc₃[a ∙ b]

= [a ∙ c][ib₁+ jb₂+ zb₃] – [a ∙ b][ic₁ + jc₂ + zc₃]

= (a ∙ c)b - (a ∙ b)c

Larson, Ron, Robert Hostetler, and Bruce H. Edwards. Calculus: Early Transcendental Functions. Maidenhead: McGraw-Hill Education, 2007. Print. 772, 789, 797.