Benoit Mandelbrot, Mathematician


The mathematician Benoit Mandelbrot (1924 – 2010) is best-known as the father of fractal geometry. He was born in Warsaw, Poland of a Jewish-Lithuanian family. They moved to France in 1936 to follow his uncle and escape Nazism, and Mandelbrot never received a formal secondary education. He was largely self-taught. He studied in France during World War II, and later moved to the United States. He married Aliette Kagan in 1955 and they had two children. In the 1950s he worked both in France and in the US. He worked for 35 years for IBM in New York and Massachusetts, and later taught at Yale in Connecticut. In 2010 he died of pancreatic cancer in Cambridge, Massachusetts.
Mandelbrot’s father traded clothing, his mother was a physician and both his uncles were mathematicians. In France, the war and the need to survive often kept Mandelbrot away from school. Nevertheless, he had an uncanny ability to visualize mathematical questions. He would solve problems with leaps of geometric intuition rather than using the “proper” techniques of strict logical analysis.

One of his uncles, Szolem Mandelbrojt, had a strong influence on the young Mandelbrot. Szolem had become an “intellectual refugee” from the academies in Poland that were influenced by Waclaw Sierpinski. He moved to Paris and became part of a group of French mathematicians who believed in “pure mathematics”, known for its rigor and abstraction, as opposed to “applied mathematics”, which concerns applying mathematics to other fields. Mandelbrot reacted against his uncle; he was a strong advocate of applied mathematics. Writing in 1987, Mandelbrot said, “While I was fond of my uncle, many aspects of his story somehow repelled me intensely. My uncle, in turn, always felt that I had squandered my intellectual gifts. Lately, however, it is becoming clear to me that in some essential ways we are alike.” This rift with French academics, where the logic and rigor of pure mathematics were strongly ingrained, eventually caused Mandelbrot to leave France for the US and become an “intellectual refugee”, just like his uncle had been.

Working for IBM, Mandelbrot was allowed the freedom to study many diverse fields. He made a conscious effort to become an expert in various unrelated areas, making contributions in fluid dynamics, information theory, cosmology and the behavior of stock markets. Looking back in 1987, he wrote: “…certain problems than others had the same “taste” to me… I always moved in hot pursuit of a technical problem that was congenial because its “taste” was the same as that of a problem I had met elsewhere and had liked.” He began to notice similar patterns in these various disciplines. When looking carefully into the detailed data, he noticed that no matter how closely he looked, there was always more detail. Also, there was a self-similarity in the patterns at different levels of magnification.

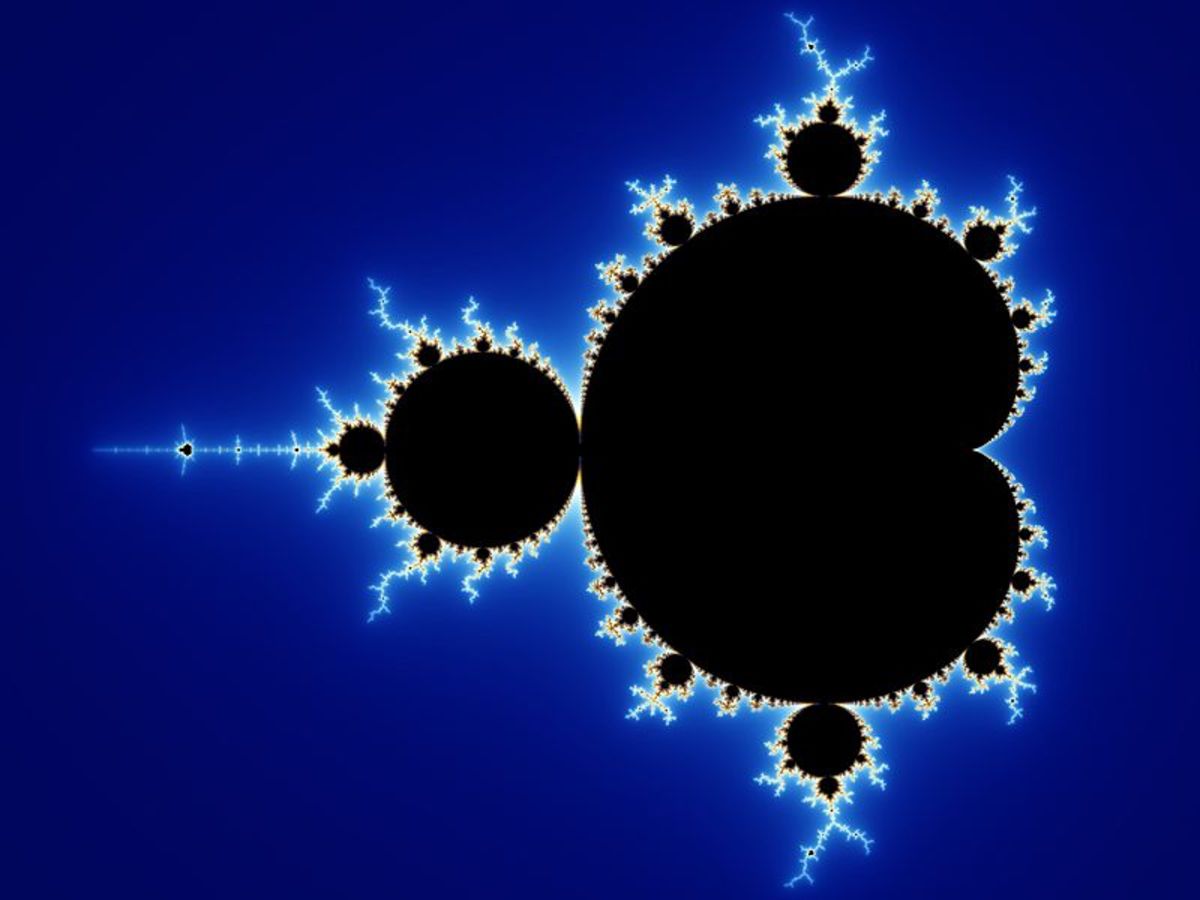
The achievements Mandelbrot is best-known for today are the discovery of the remarkable and elegant Mandelbrot set and the development of fractal geometry. Fractal geometry stands in contrast to classical Euclidean geometry, which involves smooth shapes like triangles, circles and spheres. Fractals are rough, and more closely describe the shapes found in nature. The defining works are “How Long is the Coast of England?” in 1967 (answer: “it depends”) and “The Fractal Geometry of Nature” in French in 1975. In 1980 Mandelbrot explained the term ‘fractal’, which he coined: “The word is related to the Latin verb frangere, which means “to break.” In the Roman mind, frangere may have evoked the action of breaking a stone, since the adjective derived from it combines the two most obvious properties of broken stones, irregularity and fragmentation. This adjective is fractus, which led to fractal.”

This quote from an address by Peter Clark describes some unsolved geometric problems from the early 1900’s. (Note the mention of Sierpinski, ironically the same Waclaw Sierpinski who was part of the reason his uncle had left Poland.) --
“…Now lurking about so to speak in the undergrowth of that achievement lay certain very extraordinary geometric objects indeed. To all at the time, they seemed strange, indeed rather pathological monsters. Odd indeed they were, there were curves - one dimensional lines in effect - which filled two dimensional spaces, there were curves which were well behaved, that is nice and continuous but which had no slope at any point (not just some points, ANY points) and they went by strange names, the Peano Space filling curve, the Sierpinski gasket, the Koch curve, the Cantor Ternary set. Despite their pathological qualities, their extraordinary complexity, especially when viewed in greater and greater detail, they were often very simple to describe in the sense that the rules which generated them were absurdly simple to state. So odd were these objects that mathematicians set about barring these monsters and they were set aside as too strange to be of interest. That is until our honorary graduand [Benoit Mandelbrot] created out of them an entirely new science, the theory of fractal geometry: it was his insight and vision which saw in those objects and the many new ones he discovered, some of which now bear his name, not mathematical curiosities, but signposts to a new mathematical universe, a new geometry with as much system and generality as that of Euclid and a new physical science.”
“Being a language, mathematics may be used not only to inform but also, among other things, to seduce.” – Benoit Mandelbrot, 1977.
Find out more:
Mandelbrot's home page at Yale University
Biography at the University of St. Andrew's, Scotland
Fun and easy program for zooming into the Mandelbrot set: fractalposter.com