What Are Some Common Mistakes on Proportions and How Can You Fix Them?
Units!
Oftentimes we use a proportion to help us solve a conversion. For example, we know that an inch is roughly 2.54 centimeters. If we have measured 5 inches, how many centimeters is that?
To figure that out, we need to make sure that we set up our ratio. But does the order of the numbers have to be consistent? Yes, it sure does. If I set up one side of the proportion as 1 inch / 2.54 centimeters, I better have the inches on the top and the centimeters on the bottom. Since we were given inches and I am trying to find out the centimeters, the other side of my proportion has to be 5 inches / x centimeters. So the problem altogether must be 1/2.54 = 5/x. Cross multiply to get x = 5*2.54 = 12.7 centimeters. We made sure not to mix up our units, and our answer makes sense because we had more than one inch and therefore our answer should be more than 2.54 centimeters. We could had flipped our ratio so it was 2.54 centimeters / 1 inch but then the other side of the proportion would have to be x centimeters / 5 inches. I keep the same information in the same places.
One of the main applications of proportions is cooking. Oftentimes we need to make a recipe and it serves a certain number of people, which may or may not correlate to the actual attendance. You need to only make enough to feed the people coming so you only buy enough of each ingredient in order to have no leftovers. When buying flour, the recipe calls for 3 cups of it which after adding the other ingredients will serve 2 people. You are having 10 people come over. How many cups should you get?
Well, we got to make sure we have the same information on the same sides. So I can chose to have one side of the proportion be 3 cups / 2 people and the other be x cups / 10 people. Cross multiply to get 2*x = 3*10 = 30. Divide by 2 to get x = 15 cups. Again, I kept the units together. Make sure before you solve your proportion that they match positions on both sides.
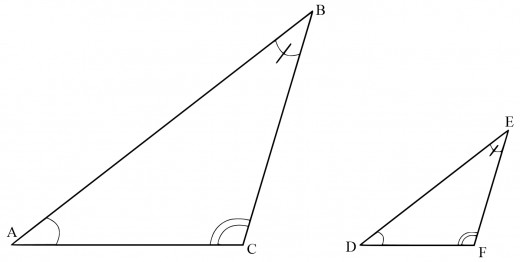
Similarity
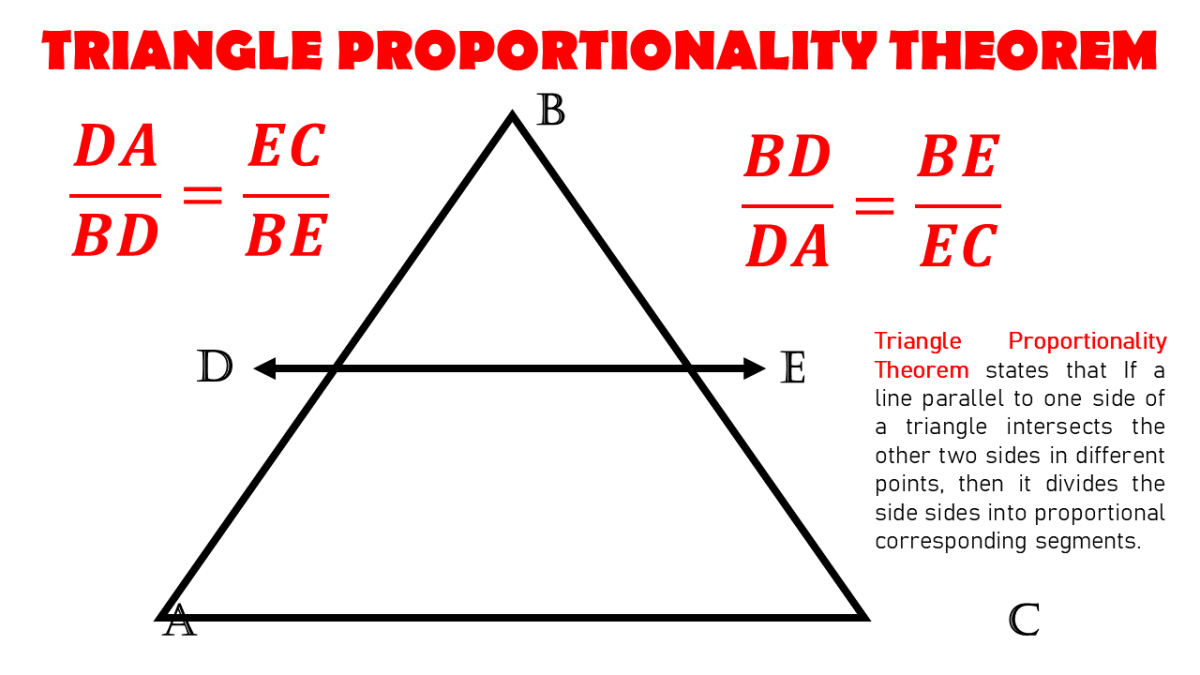
Related to the units issue is the difficulty students have with similar triangles. These are triangles that have congruent angles and proportional sides but not necessarily congruent sides. What can be difficult is setting up the proportion so that the same sides are being compared. You wouldn't want to have the short side of one triangle compared to the long side of another because they do not have a proportion between them that the other sides do. But an important fact of geometry notation will make distinguishing between the different sides much easier for us. If I have triangle ABC and DEF, the order of the letters tells me what information matches with what. Angle A is congruent to D, B to E, and C to F. And as for setting up my proportions, I could note that AB/DE = BC/EF = CA/FD. All I did was read the letters in order, and set up the proportions of the similar sides to each set.
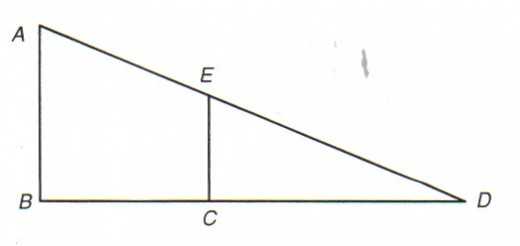
Something else that is commonly mistaken when dealing with similar triangles is making sure that when I set up my proportion I do not leave out information. This most often happens when the similar triangles share a side, like with triangles ADB and EDC. Frequently, students will accidentally use only length CB in place of DB, oftentimes because that is the only number they give us. To avoid this, remember to use the order of the letters as a guide and to use variables whenever we do not know a value. We would have AD/ED = DB/DC = BA/CE, just as before. What would be different this time would be the solving of a variable, which would be accomplished by cross-multiplying as before, then combining like-terms and solving for my variable.
© 2015 Leonard Kelley