Defining the Fractal

A fractal is a complex number map that is produced using complex numbers and has some resemblance to natural phenomena we see all around us. Fractals have an interesting history of development that really came to the fore in the early 1980s with the advent of the personal computer. This history on the other hand runs deep, incorporating some of the geniuses of mathematics in France, Germany and Russia like Cantor, Poincare, Lorentz, Mandelbrot, Fiegenbaum, Julia and others. Fractals have shown up in chaos theory, art and in animation. They developed out of number theory that combines real and imaginary numbers and have involved problems of complexity such as turbulence, orbital dynamics and population interaction.

The basic idea of a fractal map is the concept of self similarity, self affinity and sensitive dependence, which is to say that small sections of a fractal map look like the larger sections to a greater or lesser degree and that a small input can have a major impact. Fractals also tell us something of the nature of chaos, or what we once thought as chaos, but now is seen in some cases as a more complex form of order. The basis of fractal generation is pre-calculus and complex number theory. When delving into fractals, one will learn terms like recursion, feedback, bifurcation, sensitive dependence, self similarity and self affine.

Early fractal theory evolved simply enough with a geometric problem conceived by Cantor who developed the idea of breaking a line into three and removing the middle third. The two parts left on either end were further divided into three with the middle part removed and this was done repeatedly in a recursion until he ended up with a cantor set, or what we now call generally, a fractal dust. The recursive calculations can be done to the point of virtual invisibility. This is the foundation of what would later be done using pre-calculus to draw up complex maps using a combination of real and imaginary numbers. The same concept can be taken to two and three dimensions with the same result. The basic formula can be altered to create different patterns of "dust". The basic idea can be extended into the real world of clouds and galaxies that are mostly "empty space" with widely spaced solid bits.

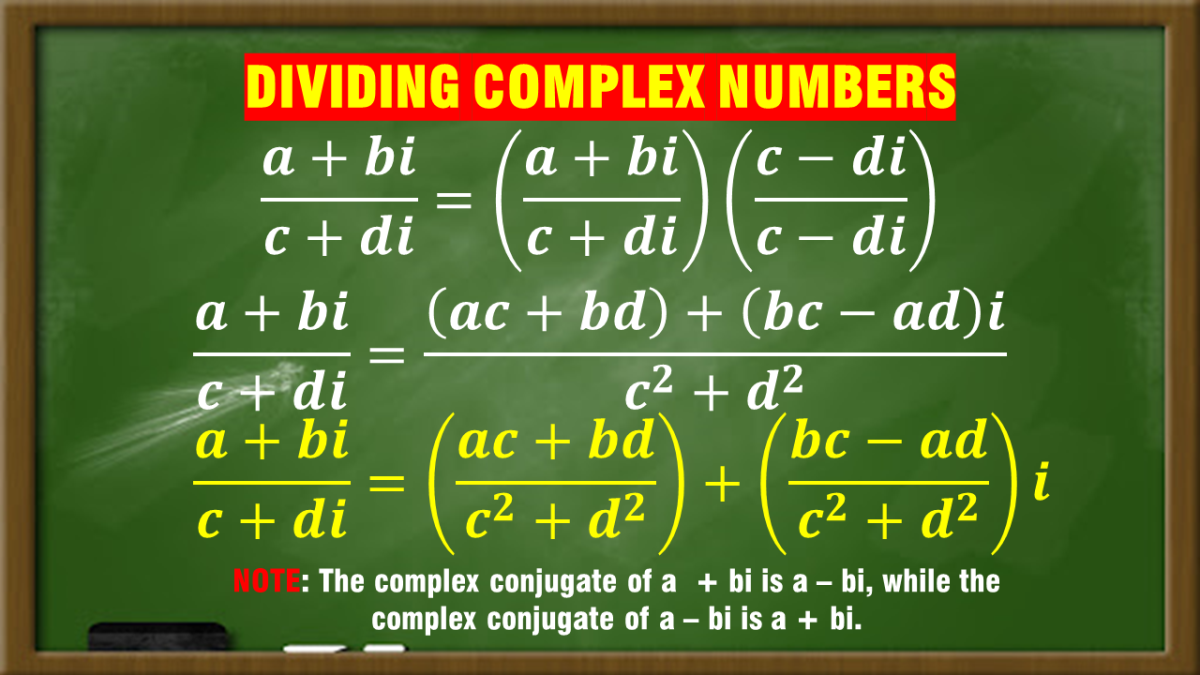
To clarify the point a little, fractals, based on real and imaginary numbers produce very complex patterns. Real numbers are what we use to count items in the physical world. Imaginary numbers are entirely founded on the square root of negative one. Finding a solution to that in imaginary numbers does not work in the real number theory, so a whole new region of mathematics called imaginary numbers result with their own set of protocols and rules. Generally, calculations have to be done separately and then combined within the confines of mathematical protocol. This even applies in computer algorithms with work arounds to arrive at true polar geometrics where fractal mapping develops through recursive calculations. The result of one calculation is taken and placed in the original equation and calculated again, ad infinitum. The more recursions one calculates, the more complex the fractal pattern emerges. The result of this is a map with three distinct regions; the region where numbers escape, where they collapse and where they just balance. With computer iterations, we can color each region of the map to determine where the numbers escape to infinity, collapse to zero or balance.

Ideas that have grown out of recent theories of chaos and complexity have led to a revolution in our view of the universe. Weather studies by Prof. Edward Edward Lorentz, for example, illustrate that the tiniest change of an input variable can lead to radical differences in outcome. The standard joke among chaos specialists is that a butterfly flapping its wings in the Amazon can influence a typhoon in the Pacific Ocean. The studies of Lorentz have been applied to real time weather; an area he first researched and found that tiny influences can have a major changing impact in short order. He ran a simple weather forecasting experiment on a computer and had to stop it after a few days. When he restarted his experiment using the last output number as his jump-off point, he found radical difference with other separate runs that had used the same input, but had run longer. The difference of a millionth part caused changes that meant totally different results within two days of forecast. He even tried starting the experiment from scratch using very slight differences in initial numbers with the same divergent results in short order. This became known as sensitivity to initial conditions. These differences could be small to the point of invisibility.

The problem he uncovered really gets unpredictable when non repeating; non ending fractions called irrational numbers are incorporated. In real number theory, there are at least as many irrational fractions as there are rational ones. This is a separate problem that has yet to be rigorously proven. What we have proven with irrational numbers is that nothing is ultimately totally predictable, as we have to round off the numbers somewhere, which leads to inaccuracies down the road and in fairly quick order. Even fractions calculated to a million places to the right of the decimal point add only a day or so of accuracy in something like weather prediction.

Among the more interesting features of fractals is something called the strange attractor. Among strange attractors are ideas worked out by Poincare on orbital theory. This single fact tells us that chaotic orbits in real space time, such as that of Earth crossing asteroid Toutatis 4179 are hard to predict with absolute accuracy. Poincare first mapped out complex orbits by studying an adaptation of the N-bodied problem and found orbits within orbits in a complex, non-repeating fashion. This type of orbit is typical of an asteroid or comet that is easily influenced by all the planets. It is also true of the planets on one another, only less so. A cross section or a Poincare orbit is study in complex pattern that is a true fractal. Strange attractors pop up everywhere, like interactive population studies. When these are mapped geometrically, they take on a characteristic butterfly appearance.

Strange attractors answer the question as to why cycles never repeat themselves exactly, but approach such similarity so as to be almost exact. The concept of the eternal return is conditioned by the strange attractor. In orbital dynamics with all the planets interacting in a complex gravitational and magnetic dance, an individual planet will never be in exactly the same orbital position twice after a complete orbital cycle, but it will come close, sometimes very close. Strange attractors pop up in all sorts of places. In fact, strange attractors define how "self affine" structures like clouds and snowflakes will look similar, but no two will ever be identical. Such is the confounding complexity of the cosmos.

Benoit Mandelbrot is considered one of the fathers of modern fractal theory and has a fractal named after him; the famous Mandelbrot set that most people are aware of when the word fractal is stated. Deep exploration into the Mandelbrot set reveals a fantastic world of myriad self similar, yet different patterns. The Mandelbrot set is based on real and imaginary number mapping and this has a whole host of other sets such as the Julia set.

Mitchel Feigenbaum was a German mathematician whose name loosely means fig tree and the fractal he found looks remarkably like a tree. His contribution is bifurcation that shows regions of order intersperses with chaos. Feigenbaum found a new irrational fraction 4.6691092..., a non repeating; non ending fraction named after him that serves as the end point of bifurcation to complete chaos.

Fractals emulate nature by mapping cratered worlds, lightning, snail shells, rivers and things like fern leaves and trees. These are the ones that are most interesting as they are accurate representations of the real items in the natural world. There are fractals that have no resemblance to anything in the real world and are positively alien in appearance. The phenomenon of fractals is growing each day and spills over into the movie industry in backgrounds and animation. Fractals have an intimate link to chaos and complexity theory that has significant impact on current thinking.

The discovery of Chaos and Complexity have increased our understanding of the Cosmos and its effect on us. If you surf the chaos regions of the internet, you will invariably encounter terms such as "sensitivity to initial conditions," "self similarity," "self affinity," "strange attractors," "feedback," "phase space," "horizon of predictability," "vanishingly small," "fractal dimensions" and so on. Each of these are easily understood, but the ramifications of these ideas have lead to a revolution in our view of the universe and as a consequence, provides a direct link to the understanding as to how each part of the cosmos interacts with the rest, including us upon the Earth as individuals and as a collective whole.