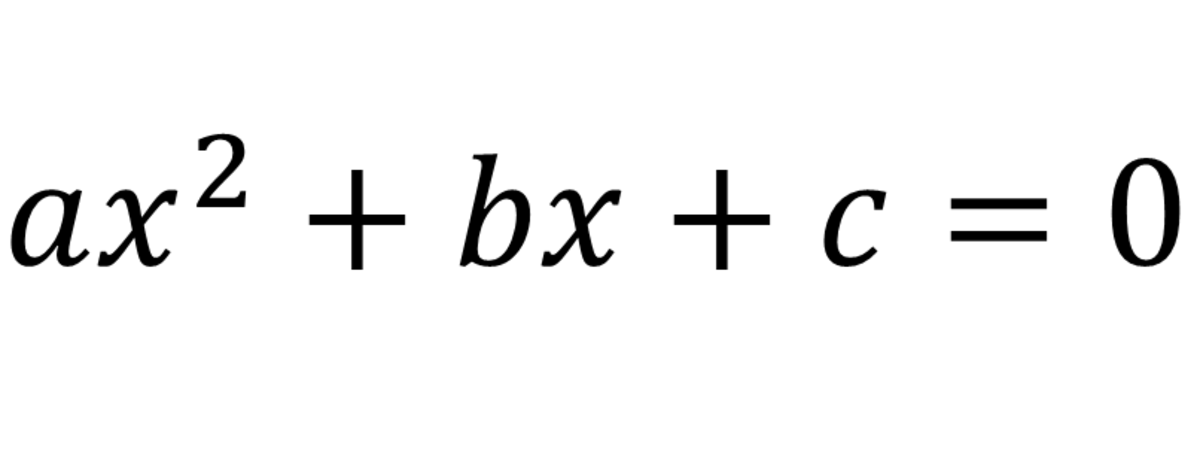Equation Solving by Regula Falsi and Bisection Methods
Here we discuss the two simplest methods, Regula Falsi and Bisection, to solve just about any equation.
About Non Linear Equation Solving
In many practical situations it is required to solve a number of very complicated equations which cannot be solved by simple knowledge of algebra. In such cases it is very handy to use a non linear equation solving technique like Regula Falsi, Bisection method, Newton Ralphston method or Secant method which can find the solutions of a very large number of algebraic equations.
However, these methods take an iterative approach by which they start with an inaccurate estimation and progressively approach the actual root to the desired amount of precision. Needless to say this involves a large amount of computing and are not at all conducive to hand solving. The easiest way to use these non linear equation solving techniques is to perhaps write a computer program to suite your requirements.
Another important concept regarding non linear equation solving is that they (theoretically) require infinite number of iterations to converge to the root exactly. So, it is essential to specify the tolerance in every case.
How does the Bisection Method Work?
In the bisection method you are required to start with an interval which contains a root of the equation, f(x)=0. The easiest way to be sure that the interval [a,b] contains a root (say p) of f(x) is to check f(a)*f(b)<0.
Then by the bisection method, it is assumed
p1=(a+b)/2
again we check if the root lies in [a,p] or [p,b] and accordingly shrink the interval [a,b]. And as before p2 is found and so on. We go on shrinking the interval until the two end points coincide (to the prescribed degree of tolerance) and that is the required root.
How does Regula Falsi Method Work?
Regula Falsi method uses an exactly similar approach except for the fact that the values of pi are calculated as
pi=b-f(b)*(b-a)/(f(b)-f(a))
where a and b are the end points of the interval containing the root during the ith iteration.
It may be worth noting that the value of pi is nothing but the x intercept of the line joining (a,f(a)) and (b,f(b)).
Important Notes on Regula Falsi and Bisection Methods
It is important to keep the following things in mind while performing the Regula Falsi or Bisection Methods:
- These methods are linear in nature and will in general be slower than higher order methods like New Ralphston (quadratic).
- These methods will fail if f(x) has repeated roots. (eg. (x-2)2 cannot be solved by Regula Falsi or Bisection Methods)
- Regula Falsi or Bisection Methods assume there is only 1 root in the given interval and in case of multiple roots it will converge to any one of the roots.
- These methods can only deal with intervals having odd number of roots. So choose your intervals accordingly
Further Reading
If you wish to know more about bisection or regula falsi methods (accelaration techniques, most efficient tolerance criteria) as well as other numerical methods then it is essential to have a comprehensive guide book which will explain to you the main concepts without delving too much on the high end mathematics used to devise such methods. Personally, I will definitely suggest A Friendly Introduction to Numerical Analysis by Brian Bradie for any new student of this field as the book lucidly covers the most popular numerical methods and provides you with a very good feel of the subject by not only demonstrating every technique through illustrative example but also by tackling interesting real life problems.