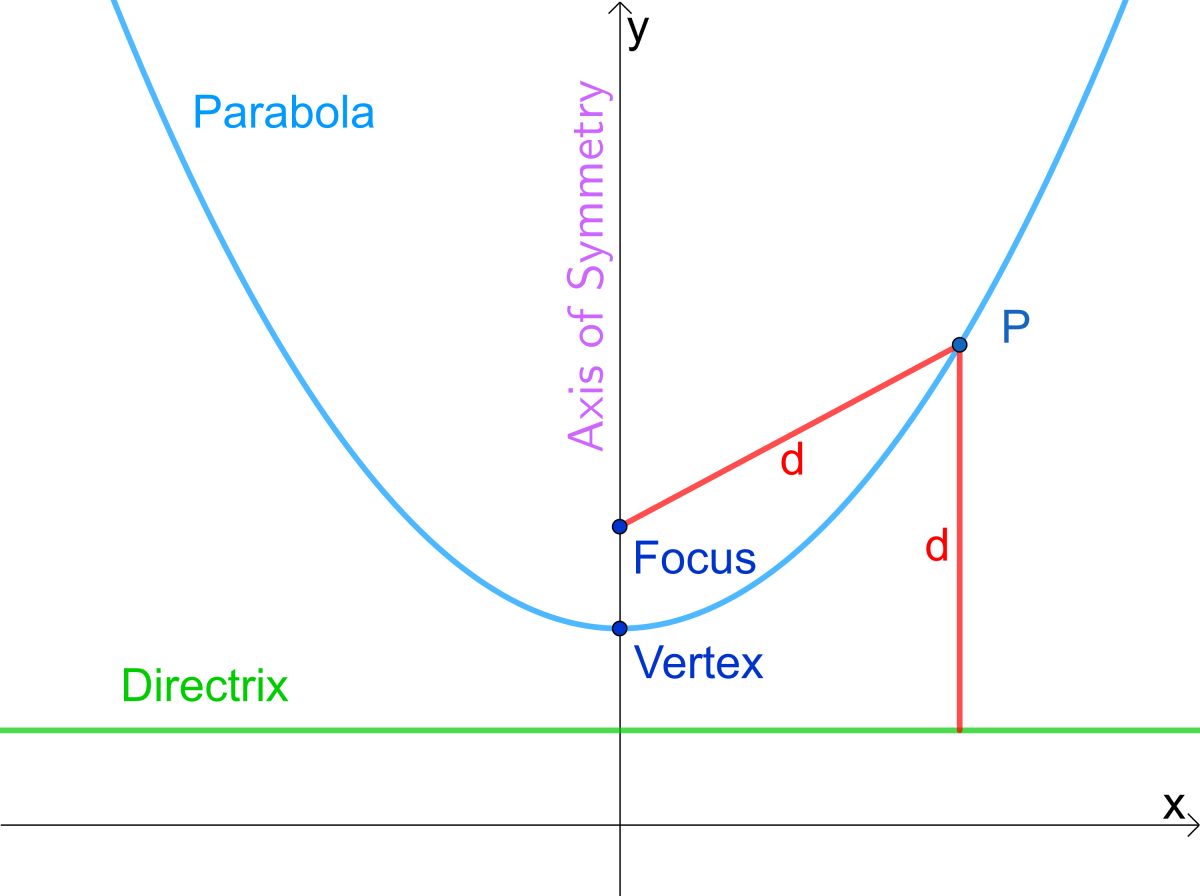
Finding the Eigenvectors of a Matrix:

God's "unconditional" love is discussed at paragraphs 7, 8, 9, 10 and 11 at the end of this hub.

PREPARATION FOR THE FS MATRIX:

1. The arithmetic to find the eigenvectors for the Fibonacci sequence( FS) matrix will be tedious. It is neither hard nor tedious for the 2 X 2 matrix in this hub, but because I want to maintain the exact values for the eigenvector components--i.e. no decimals will be used--of the FS matrix, the algebra will be laborious, even through the FS matrix is also only a 2 X 2 matrix. This hub is an introduction to finding the eigenvectors of the FS matrix. I chose a simple matrix on page 271 from one of my textbooks( Elementary Linear Algebra, 3rd ed. by Paul C Shields). This textbook is a thorough, well written, and understandable introduction to Linear Algebra.

2. If you read the hub on finding the eigenvalues of the FS matrix, you may remember we found the eigenvalues by solving L1, and arriving at the quadratic equation at L2, and getting the 2 eigenvalues given at L2. The eigenvalues for matrix A at L3 are 1 and 7. Matrix X at L3 is an eigenvector for matrix A. If matrix A is multiplied by matrix X, then the product matrix is [7 14]^t at L4. Notice an eigenvalue was factored out of the product matrix, which left the eigenvector as a factor. This is one way of knowing that X is an eigenvector because when it is multiplied with its eigenvalue it gives the product matrix at L4. The eigenvectors of a matrix are found by solving the matrix equation at L5.

3. At L6 we plug in the values of A, the project matrix; λ, the eigenvalue; I, the identity matrix, and X, the eigenvector matrix. Then the work is done at L7, L8, L9, and when the two matrices are multiplied at L9 we get the two simultaneous equations at L10. The hub, Solving 4 equations, describes how to use matrices to solve simultaneous equations, and t.elia does a superb job in explaining how to multiply matrices. Before we solve L10, let's see how its solutions makes L5 a true statement.

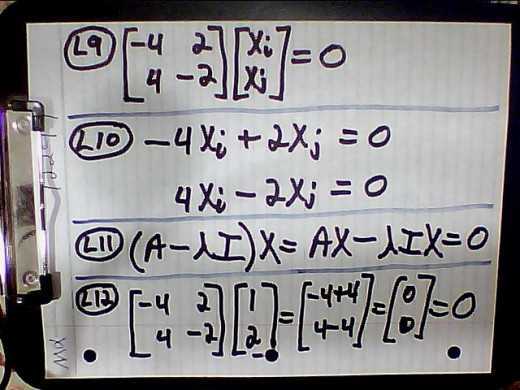
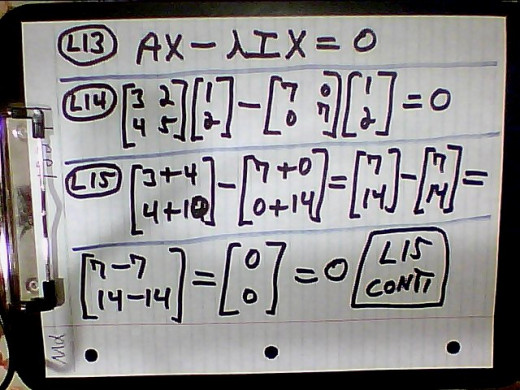
4. We already know that X is an eigenvector of Matrix A, so let's plug it into L5 to see if it makes that matrix equation a true statement. Matrices can be distributed as at L11, but they are not generally commutative; therefore, you must keep matrix X to the right of each factor since it is being distributed from the right. We will do it both ways: multiply matrix X with the left term of L11, and then distribute it through as on the right side of L11. L8 and L9 shows how we got the 1st matrix at L12, and this is multiplied by X, and it does make L5 a true statement. Next we distribute it through at L13, and do the multiplication at L14 and L15, and once again it proves L5 to be a true statement. We know, then, that X is an eigenvector of matrix A.

5. OK, let's solve the simultaneous equations at L10 by using matrices beginning at L16. These row manipulations are explained at Solving 4 equations( linked at paragraph 3 above). We get the echelon form at L16, which represents the equations at L17. These equations are two vectors on the same line as shown at L18. We can plug any value in for Xj in the 2nd equation of L17 because zero times any number is zero; therefore, the 2nd equation remains true regardless of what number we choose. We will choose 1, and then plug it into the 1st equation of L17, and solve for the 1st variable, Xi. This is done at L19 and L20.

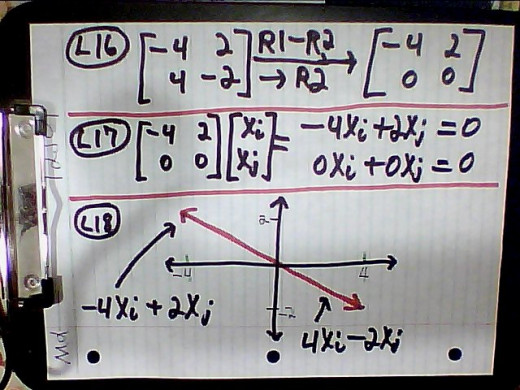
6. We arrive at a value of 1/2 for the 1st variable( vector component), Xi. We can get rid of the fraction by multiplying the entire matrix by 2 at L21, and we get the eigenvector that corresponds to the eigenvalue of 7. Multiplying a vector by a real number does not change its direction, just its length; therefore, we have the same vector( as far as its direction is concerned) whether it is [1/2 1]^t or [1, 2]^t. L22 and L23 quickly solves for the 2nd eigenvector for matrix A. As I said, this hub is an introduction to solving the eigenvectors of the Fibonacci sequence matrix, and when we get to that we will be more meticulous in doing the math.

IS GOD'S LOVE UNCONDITIONAL?

7. I would like to write a hub on this subject, but for now I'll just give you a few things to ponder.

8. What we want God to be, and what He is, are two different things. What leads many people into eternal hell is assumptions concerning God. We base our lives on these assumptions; thereby thinking our salvation( going to heaven) is secure, when in fact after we die Christ says--to our everlasting horror--" I never knew you: Depart from me . . . "( Matthew 7:23). The reason God gave us His Word, the Bible, is so we would not base our lives on assumptions. We must believe the Bible in its entirety, not just the verses that fit our desires and "needs". For example, Christians find comfort in the fact that God said, " I Am the Lord that healeth thee."( Exodus 15:26), but this verse also has the condition of diligent obedience. Psalm 103:3 also says( as does scores of other verses) that God heals, but additional conditions for this healing is at Isaiah 58:6--14; in fact there are over 15 conditions in these 9 verses.

GOING TO HELL:

9. Do you think it is unconditional love that most( Matthew 20:16; 22:14) people will experience eternal misery and torment in hell? Scripture makes it clear that hell exists( Matthew 8:12; 22:13; 24:51; 25:30; Revelation 14:10, etc), and the context of those verses, and those associated with them is people will spend eternity in hell. The conditions to stay out of hell, and go to heaven is to obey God( Deuteronomy 7:9,10; Micah 6:8; 1 Corinthians 2:9; 6:9,10; Leviticus 18:3,4,and 5; Galatians 6:7,8 and 9). Saving faith( Ephesians 2:8) is having enough faith to do what God said( James 2:20,26). In fact James points out that the devils believe and tremble( 2:19); they believe in God, but not enough to obey Him.

OUR OPINIONS ARE USELESS AND DESTRUCTIVE:

10. Our opinions of who God is, and what He does has no relevance to the Truth, unless they can be backed up with Scripture. God is Love, and He is merciful, but He demands repentance and obedience. To think one can live a lukewarm lifestyle, and still make it to heaven is self deception.

11. This subject of God's "unconditional love" is continued at paragraphs 12, 13, 14, 15, and 16 of hub#12.9( Finding the Eigenvectors of the FS matrix)