Kalam Cosmology and the Infinite

In Medieval times the argument that the world was eternal was focused on demonstrating that an actual temporal infinity was possible. On the other hand Creationists offered the basic arguments why the concept of an infinite past was absurd. Their argument was based on these facts: a) the infinite cannot be traversed, b) the infinite cannot be added to, and c) infinite series cannot be put into ratio with each other.

The primary source of this argument is found in Kalam Cosmology in an effort to demonstrate that since an actual infinity leads to absurdities the world must be finite and created. The Muslim school of thought, called the Kalam, was interested in cosmology and developed many arguments against temporal regress. The Kalam school of thought developed in the Middle Eastern nations in 9^th to 12^th century and in Muslim Spain from thr 10^th to 12^th century. A philosopher who followed the Kalam was called a mutakallimun. The mutakallimun adhered to the notion of creation ex nihilo and were against the Aristotelian notion of the eternity of the word.

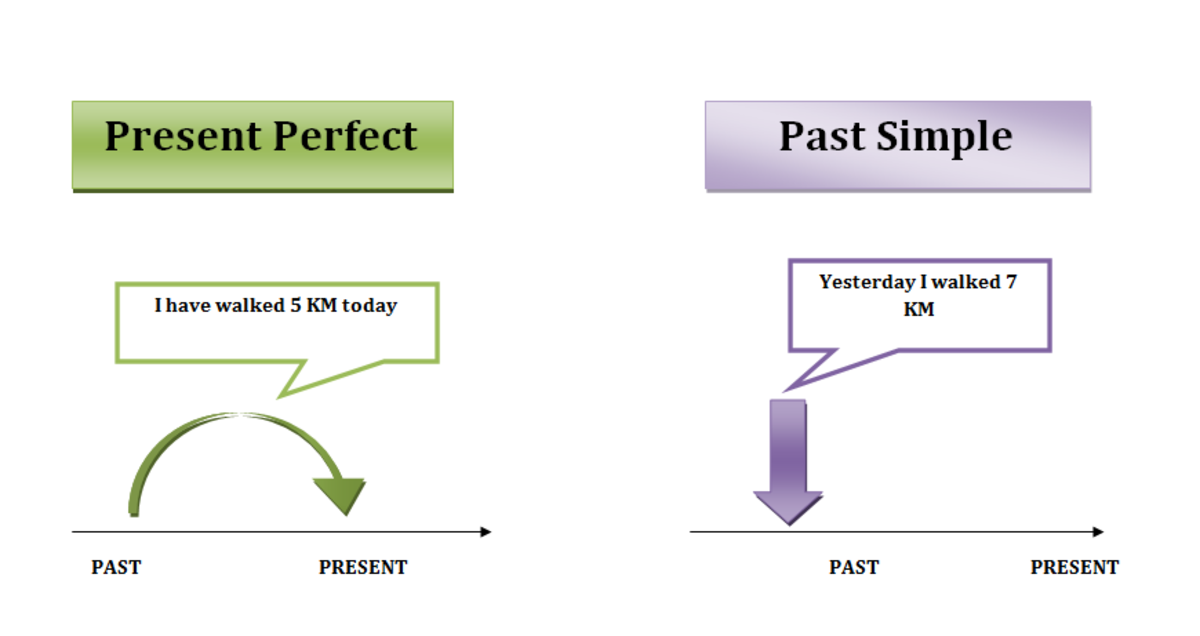
One feature of the infinite is it is boundless and the traversal of it seems impossible. The series of whole numbers is a series that we know is infinite and it is precisely this that makes us distinctly aware that we would be unable to count all the numbers in the series and thus unable to reach the end. When a series is instantiated in time, the problem becomes we find we are unable to reach the present. Yet clearly we are in the present, therefore the past is finite.

There is the temptation when faced with the problem of traversing the infinite to state that due to the infinite divisibility of time and space we traverse the infinite every passing moment and with every step. However, just because time is infinitely divisible does not mean it is actually ever infinitely divided. Time can be potentially divided infinitely but is never actually divided so.

The Islamic philosopher Ishaq al-Kindi (800-873) puts the argument of traversing the infinite as if the past were infinite “then before every segment of time there would be another segment, ad infinitum. But in that case no time could ever be reached. For [the duration] from the infinite past to the given time would be equal to the duration ascending back the given time… to infinity… If something is infinite, its interval cannot be traversed.” (Davidson, p.107). Al-Kindi states that the duration from the present moment back into infinity is the same duration from past infinity to the present moment. His reasoning is that if we know the duration from past infinity to now, then likewise we know the duration from now back into infinity. This would make the infinite finite, as it would have known limits. The problem is that to select any given moment we must know that moment in relation to the past time before it not of the relation t the present moment of time. Al-Kindi appears to believe as if there is an infinieth point in the past, and therefore if we know how far the duration from the present to that point is then indeed it is a finite duration. Since there is no such point we do not know the duration from now into an infinite past. In fact any given moment of the past we can locate to the present would be a finite duration. Philosophers such as Nazzam (775-845) said that which has no beginning could have no end, and if the present is the end of the temporal series then the past must be finite. The Jewish philosopher Saadia (882-942) states that it is impossible to mentally traverse the infinite backwards and likewise it is impossible for existence to traverse it actually forwards.

Aquinas (1225-1274) brings up a response that states the entire infinite interval does not have to be traversed, only a finite portion. For “’Though the infinite is not [traversed] when simultaneous and actual, it can be [traversed] when in succession [and in potentiality], since any part of such infinites taken is finite,’” (Wolfson, p.461). Also according to Aristotle “an infinite cannot be traversed refers only to a continuous infinite extension which has neither beginning nor end, but in the case of an infinite by succession… means that ‘one things is always taken after another, and each things that is taken is always finite,” there are parts which are traversed.” (Wolfson, p.461-2). Between any two given days the interval is finite and therefore whatever day in the past we choose to the present there are only a finite number of days to traverse. Basically the whole infinite is not traversed because the infinite is successive in nature and traversed term by term. However, if within the series there are infinitely distant events then there would remain the problem of traversing an infinite interval.

The idea that you cannot add to an infinite collection seems to arise from the idea that the infinite is the greatest number instead of greater than any assignable number. The intuition then is that if you have a series that is infinite and add one more member you get something that is greater than the infinite. However, if we take the infinite to be greater than any assignable number, when we add to the infinite it remains infinite. The series of whole numbers would be construct an actual infinite because it has no last term and it contains all the whole numbers and it seems there are no whole numbers to add. The reasoning is that if you add to any magnitude, including an infinite one, is should then increase in size. Therefore if by adding to the infinite it fails to be increased then it is seen to be absurd. However, now we would say we can add to this series simply by starting over (1,2,3.…1,2,3). By adding to the series we have not created something greater than the infinite because the infinite is greater than any assignable number, and therefore we can continue to add to this series without creating something greater than the infinite.

Al-Kindi demonstrates the impossibility of adding to the infinite based on some simple principles. If two bodies are added together then the body created will be greater. If two finite bodies are combined the result will be finite. Now if you have an infinite magnitude and remove a finite portion the result will either be finite or infinite. It cannot be finite, “for when the subtracted portion is restored, the total, as the sum of the two finite magnitudes , would also be finite.” (Davidson, p.108). It cannot b infinite and yet “fail to be increased when the subtracted portion is restored; for every magnitude undoubtedly becomes greater when another magnitude is added to it.” (Davidson, p.108). Nor “could the remainder be infinite and yet… be increased through the restoration of the finite portion, for ‘there cannot exist two infinite magnitudes of the same type one of which is greater than the other.’” (Davisdon, p.108). Al-Kindi further states that the smaller infinite would be equal to part of the whole. He believes if the smaller infinite is contained within the whole infinite then the part would have limits and thereby cannot be considered infinite. The smaller infinite also cannot be equal to the combined infinite, because that would result in the whole being equal to its parts. What is important to note here is that the principle the whole is greater than its parts does not seem to be valid in regards to infinite qualities. Al-Kindi would like to hold to this common sense principle and therefore it is impossible that the parts of the infinite could be equal to the whole.

Saadia also states with each passing day the infinite past would be added to and as the infinite cannot be so increased past time must be finite. He phrases it as each passing day increases the past it diminishes the future and whatever admits increase and diminution is of finite power. He mentions the future because he wants to show the future is also finite. This is brought up by Shahrastani as he states “’if we took off a year from the past and added it to the future, then the past would be decreased and the future would be increased, even though they are both equal in infinity, whence it would result that the more would be equal to the less.’” (Wolfson, p. 424). Saadia’s point that what is capable of increase and diminution is finite applies to potential infinites, since no matter how much increases the series it remains finite.

The argument that infinite series’ cannot be put into ratio often occurs along with the argument that the infinite cannot be added to. The argument is usually illustrated by the revolutions of various celestial spheres found in Medieval astronomy. If the universe is eternal then each celestial sphere has completed an infinite amount of revolutions. Yet the Sun will have thirty times more revolutions than Saturn every year, which implies the Sun revolutions would be thirty times greater than the series of Saturn revolutions. Not only is each infinite series added to by additional completed revolutions but one infinite series is thirty times greater than another infinite series. The puzzle that arises from that is that while both series being compared are equally infinite, one is at the same time greater than the other.

Ghazali (1058-1111) notes that if you wish to side step this argument by claiming the sphere’s revolutions cannot be numbered, there is still a clear ratio between then that must be acknowledged. They do stand in a numerical relation, even if you cannot compare part to part. He states “’just as the number of revolutions of Saturn is infinite so also the revolutions of the Sun is infinite, despite the fact that the former is one thirtieth of the latter.’” (Wolfson, p.422). Yet there remains the claim that they are both infinite and cannot be compared, because neither series is complete. The series is complete in the sense that the present would be the end of the series. It is not complete, however, in the sense that it is open-ended or infinite in the past. Since we cannot establish a beginning we are unable to begin to compare parts. This is precisely the point raised by Averroes. Averroes claims that given the infinite revolutions of any sphere are not a competed whole with definable limits, there can be no ratio established. Averroes being aware that Aristotle stated “’the infinite is that of which, however much on has taken, there is always more to take, whereas that of which there is nothing more to take is complete and whole.’” (Wolfson, p. 431) and the infinite unlike a completed whole lacks at least one end or limit. If the series were finite then indeed we could make the claim one series is greater than another, as we would simply compare each part of both series from the beginning to the end. There cannot be a comparison in sizes of the infinite series if neither is a completed whole. Two infinite series cannot be compared part-to-part, since it makes no sense to speak of parts when there is no completed whole. Alfarabi (870-950) further explains that past motions continue to exist in the imagination “since they do not exist together in actuality, they cannot properly be enumerated and therefore are not affected by the absurdity of an actual infinite number.” (Davidson, p.128). As Ghazali notes there is a ratio present regardless of whether we can compare the series’ part-to-part. This is because we are aware that in the same span of time one sphere makes thirty times more revolutions that the other, and this correlation enables us to state that one series is thirty times greater without comparing each part of the series from a determined beginning. However, if we want to compare sizes of the infinite series we cannot do so based on finite notions of wholes and parts, nor can we do so by counting each member individually.

As we can see there have been many problems that complicated the idea of the infinite and an infinite collection. The problem of traversing the infinite seems to imply that we would have to traverse an infinite amount of moments to reach the present moment. If a series has infinitely distant events then we are confronted with this notion of traversing an infinite to reach the present. But if there are no infinitely distant events then we find that any point in the past to the present is finite. The fact that an infinite collection of moments has receded into the past seems to suggest that all that is traversed is finite terms successively instead of an infinite progression of moments; each moment requiring the one previous to it, and so on ad infinitum. There seems to be no easy answer to the notion of traversing the infinite as it arises from our awareness of the infinite being endless. As we are aware of our inability to count to infinity we find it incomprehensible a series with no beginning can have an end.

Secondly if the infinite is described as the greatest number conceivable then it seems odd that we could add to that creating something greater. The infinite should be described as greater than any assignable number and therefore adding to an infinite does not create something greater than an infinite.

Medieval ideas are focused on the axiom the whole is greater than the part, which clearly hold for finite collections and causes absurdities when applies to infinite ones. If the world is eternal then the sphere of the Sun would have made an infinite amount of revolutions. However, the Sun would have completed an infinite amount of revolutions a hundred years ago as well. That smaller segment is infinite just as the whole series is. Reason tells us that the whole series should be greater than the part. However, the subset is this case is not a completed whole, and neither is the entire set, thus both being infinite they can be considered equal in size. Medieval thinkers considered the notion that in an infinite series the whole is equal to the part is absurd and paradoxes thus arise from accepting it. The axiom can be discarded here because infinite collections, unlike finite ones, are not intervals that construct finite wholes with two ends and so the axiom does not apply.

If we take the sphere of the Sun and the sphere of Saturn both sets of revolutions separately create their own infinite series and operate under a ratio of one to thirty. It can be replied because neither series has a first term we cannot establish a ratio, but medieval thinkers believed the Sun completed thirty more revolutions a year. It s reasonable to conclude the series of Sun revolutions is thirty times the series of Saturn revolutions. At the same time, if both series are infinite then they are also the same size. The principle the whole is greater than the part is not valid for infinite collections. A section of an infinite series can be equal to the whole series when the series is infinite. Regardless of the fact that the Sun series appears to be thirty times greater than the series of Saturn both of them construct a denumerable (or countable) infinite and therefore are equivalent.

Notes

Albert, Nikki The Tristram Shandy Paradox. 2002

Davidson, Herbert A Proofs of Eternity, Creation and the Existence of God in Medieval Islamic and Jewish Philosophers. Oxford University Press: Oxford NY 1987

Wolfson, Harry Austryn. The Philosophy of the Kalam. Harvard University Press: Cambridge, England 1976.