What Are Material Conditionals and Bi-Conditionals in Logic?
In symbolic logic, we have special types of sentences that have truth value patterns that can be extended to many other sentences and allow us to draw new conclusions. Some examples include conjunctions and disjunctions, which contain “and” and “or.” These link two sentences together and making “both” necessary to be true (“and”) or just needing one or both of them to be true (“or”). We can also negate a sentence by taking its opposite truth value as the overall value we are seeking. But what about statements that require a condition to be true for a conclusion to be true also? That is what do we do with “if A, then B?” What do we call this?
Material Conditionals
This is known as a material conditional (Bergmann 41). We can think of “if A then B” as being paraphrased as “either it is not the case that B or it is the case that A." This is true because since A implies B we know that if B does not happen then neither does A but if A happens then B follows. We can symbolize this as ~ B v A, with the "~" a negation and “v” meaning “or.” We can also create a new symbol, an arrow, to signify an “if … then…” statement represented above. This looks like A -> B, though some textbooks use a horseshoe “⊃” instead of an arrow. A, the “if” sentence, is known as an antecedent while B, the “then” sentence, is known as the consequent. The truth table for conditionals is easy to remember: the conditional is false only if true premises lead to a false conclusion (41-2).
Now, a fair warning about paraphrasing sentences into conditionals. If you see “A only if B,” do not think that B is the antecedent just because it is after the if. In these types of paraphrases, B is the consequent and A is the antecedent, or "if A, then B." Also, when we see “A unless B,” we can paraphrase that as either a disjunction ("A or B") or as a conditional ("if A, then B"), depending on the context of the statement. Logic keeps us on our toes, as you can see, so stay alert and be mindful about what you are doing (44).
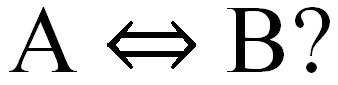
Material Bi-Conditionals
Now, if I have the conditional “if A, then B,” what happens when I switch the order of the precedent and the antecedent? Would the statement “if B, then A” be true because “if A, then B” was true? Not necessarily, for if A was false and B was true, then “if A, then B” would be true but “if B, then A” would be false. By switching the order, we got to be careful because the truth of the conditional changes. But what if both “if A and B” and “if B, then A” were true at once?
That would be known as a material bi-conditional, and can be symbolized as the conjunction of two conditionals, or (A -> B) ^ (B ->A). To paraphrase into a bi-conditional, we could go with “either both it is A and B or both is not B and that it is not A.” This is because of what we demonstrated above in the conditional section. If they both have different truth values, then one of the conditionals would be false, so both the antecedent and the consequent must have the same truth value. We can symbolize the paraphrase as (A ^ B) v (~A ^ ~B). Typically, however, we symbolize a bi-conditional as a two-headed arrow, or A <-> B, though some books may use a triple bar instead (45-6). You can also paraphrase “A if and only if B” as a bi-conditional statement.
Works Cited
Bergmann, Merrie, James Moor, and Jack Nelson. The Logic Book. New York: McGraw-Hill Higher Education, 2003. Print. 41, 42, 44-6.
- Connections Between W.V. Quine’s “Meaning and Tr...
In Quine’s “Meaning and Truth,” in particular the section "Truth and Semantic Ascent" (TSA), and Davidson’s “Truth and Meaning” (TM), the generalization of truth is discussed. For Quine, he makes use of semantic ascent, where we use... - Negations, Conjunctions, Disjunctions, and De Morgan...
In logic, De Morgan's Laws are tools that can be used to transform an argument into a new, potentially more enlightening form. We can make new conclusions based off what knowledge we have at hand. We start off with two statements that are somehow...
© 2013 Leonard Kelley








