Want To Ace GRE Math? Understand This Unique Format

If you are preparing to take the GRE and it’s been a while since you solved for x, you may be worried about reviewing “all that math.” (It’s even worse if you never liked math in the first place ….) So let me start with some good news for math-phobic test takers: Though I agree that math review is important to your GRE performance, I would wager that it is not the most critical factor to improving your GRE math score. This is partly because the level of math on the GRE is less daunting than most test takers anticipate. It’s actually about the same level of math as on the SAT or ACT exam: no calculus or even pre-calculus; only basic geometric figures; only the simplest of statistical concepts; and so on.

I rule out math review as the most important GRE test prep task for two additional reasons. The first is that test takers can often bypass more challenging math concepts by applying actual numbers – a strategy that I explore in detail in a series of test prep hubs. The hubs are directed toward those preparing for the SAT, but they are completely relevant to standard multiple-choice problems on the GRE.

The second reason is unique to the GRE. It’s a test item format called Quantitative Comparison (QC). Getting used to this format, understanding the logic behind it, knowing what to look for when solving QC problems – in my 10 years of teaching GRE prep, I have found that GRE test takers can improve their math score the most by mastering the strategies and tactics for solving QC questions. So let’s start with some key pointers about QC. Then in a couple follow-up hubs, we’ll look at applying actual numbers to QC problems – a strategy that will give you a way to efficiently arrive at the correct answer on many of these questions.

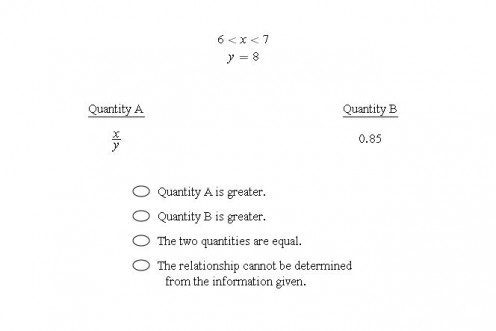
Above is a typical QC problem. It is taken from a practice test published by ETS, the same folks who write the actual GRE. One tip I regularly give my test prep students is to practice first with questions written by the test writers. This helps to ensure that the questions on your actual GRE are like the questions you've practiced.

The QC problem starts out with some information that applies only to this particular problem. It then gives two quantities, and four answer choices. Every QC problem on your GRE will have the same four answer choices in the same order. Understanding these answer choices is the first step in understanding how to solve QC problems.

The four answer choices for QC problems present the four possible mathematical relationships between between Quantity A and Quantity B. Using the example above, the answer choices mean the following:

As you might imagine, answer choice (D) is what throws a wrench in the works. Because of (D), even if I find that Quantity A could be greater than Quantity B, I still have to determine whether Quantity A is always greater than Quantity B. In fact, I like to add the word “always” to answer choices (A), (B) and (C):

(A) Quantity A is always greater.
(B) Quantity B is always greater.
(C) The two quantities are always equal.

Then there’s the language of answer choice (D). You’ve seen something like this before. Every so often, the last answer choice on an SAT or ACT math problem is “It cannot be determined from the information given.” But here is an important difference: On the SAT or ACT, “It cannot be determined from the information given” means that you can’t arrive at an answer. In QC problems, however, “The relationship cannot be determined from the information given” means, in effect, that you can arrive at too many answers!

Allow me to illustrate with our sample problem. The value of x can be anywhere between 6 and 7. So let’s put x really close to those two end points. If x = 6.0000001, then

This is less than 0.85. But if x = 6.9999999, then

This is greater than 0.85. So the relationship can change. And
that’s exactly how I would phrase answer choice (D):

(D) The relationship can change.

The language I’ve proposed for the four QC answer choices also suggests a step-by-step strategy for these problems.

1. Using one set of valid values for any variables, determine if Quantity A is greater than, less than, or equal to Quantity B.
2. Cross off the two answer choices that this first set of values rules out. For example, if Quantity A is greater than Quantity B, then I can cross of answer choices (B) and (C). I know for certain that Quantity A is not always less than Quantity B, nor are the two quantities always equal.
3. Using other sets of valid values try to get a different relationship between the two quantities.
   
   a. As soon as you succeed in getting a different relationship, select (D). You
   just demonstrated that the relationship can change.
   
   b. If the relationship won’t change no matter what values you select, then
   select the answer choice that corresponds to the relationship you keep
   getting.

By “valid value” in this strategy, I mean a value that the “rules” of the particular problem allow. For our sample problem, the rules tell me that y’s value is always 8, and that I can select values for x only between 6 and 7.

If I’m going to employ the strategy I’ve suggested, then this means that I actually have a goal of making the relationship change. So I’m going to try to think of ways to get it to change. In the sample problem, I chose values for x at the extreme ends of the range. If the relationship between the quantities is going to change, then I’m likely to observe this change when values I pick are as far away from each other as possible. Only after I’m convinced that I can’t achieve this goal – that is, once I’m confident that the relationship isn’t going to change – will I pick an answer other than (D). (Please note, like all multiple-choice tests the GRE is designed to ensure that you won’t get an inordinate number of (D)s; so be prepared to fail pretty regularly at making the relationship change.)

The strategy I’ve suggested also means that QC is a good place to apply actual numbers. If you haven’t yet reviewed my hubs on applying actual numbers to multiple-choice problems, I encourage you to do so. After you’re familiar with the technique, then read my next two hubs about the GRE, which will show how to apply actual numbers to QC problems. The procedure is similar for QC and multiple-choice formats; but there are important distinctions too, as you’ll see.