Maths help: What are co-ordinates? X axis, Y axis, across the landing and up the stairs + Fun activities you can use

What are co-ordinates?
Coordinates are a pair of numbers that describes the position of a point on a grid. To find a point's coordinates, you need to look at the numbers of the lines it sits on.
First look at the number of the line along the x-axis (the line that goes from left to right) and next the number of the line up the y-axis (the line that goes up and down).
Then you write the coordinates in brackets with a comma between the numbers like this: (x,y)
Children must learn that it is ALWAYS the x-axis coordinate that is wrote first.
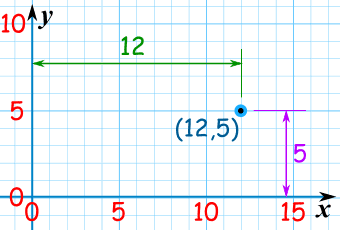
If you look at the example above then the point sits above the 12 on the x-axis. It is also across from the number 5 on the y-axis. So if we were to write down the coordinates of this point it would be (12,5).
Lets look at this in more detail:
x-coordinate:
The x-coordinate is the number that shows the position of a point along the x-axis. The x-axis is the line that goes from left to right. It can also be considered to be a horizontal line if you want to help the students with more mathematical language. So if the line is horizontal then the x-coordinate would be either above or below it - Above if it is a positive x-coordinate and if it is below then it would be a negative x-coordinate.
y-coordinate:
The y-coordinate is the nmber that shows the position of a point on the y-axis. The y-axis is the line that goes up and down. It can also be considered to be a vertical line if you want to help the students again with more mathematical language. So if the line is vertical then the y-coordinate would either be to the left or right of this line - left if it is a negative y-coordinate and to the right if it is a positive y-coordinate.
NOTE: I would start with the x and y coordinates being positive, so you are only dealing with the 1st quadrant. Once they understand the concept of the x-coordinate ALWAYS being the first number in the brackets then they can move onto all four coordinates.
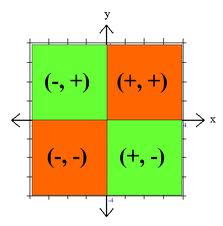
The four quadrants
The picture opposite shows the four coordinates. Once children are confident with negative numbers and the use of coordinates in that the x coordinate comes first.
1st quadrant:
Both coordinates are positive (this is the first quadrant that all children should learn first.)
(x,y)
2nd quadrant:
X-coordinate is negative and the y-coordinate is positive.
(-x, y)
3rd quadrant:
Both the x-coordinate and the y-coordinate are negative.
(-x,-y)
4th quadrant:
The x-coordinate is positive and the y-coordinate is negative.
(x,-y)
Negative coordinates
The way I explain negative coordinates to the children is to have a number line on the board and ask them to label the numbers below the 0 mark (This should be easy as they should have already tackled this in previous lessons.)
I also do it for a number line which stands vertical to see if they can do that.
Once this is done I show them a grid with all four coordinates and ask them what they notice.
Basically negative coordinates are numbers which sit below the zero mark. This would be to the left of the x-axis or below the y-axis.
So what sort of things can I do in class to help my children learn coordinates?
NOTE: ALWAYS, ALWAYS write the x-axis number first. This is where children get things wrong. There are a few things you can do to make sure they do this like:
Lesson idea 1
Young children could be told a story - "The three bears went into the house and up the stairs ...," While telling the story you model the movement of the three bears.
Lesson idea 2
You could tell the children rule of "along the corridor and up the stairs" to help them remember. This is something that my year 7's have remembered however once they start to learn coordinates in the four quadrants then this rule doesn't quite work properly.
This idea will need to be modelled like the story idea so they know what it means. Then they should have a go at the exercise you have come up for them.
Lesson idea 3
Children should be given a shape and be asked to write down the coordinates for someone else to draw.
I did this with a year 3 class and drew out spongebob, Gary, Mr Crabs and Patric for them to have a go at. It was differentiated depending on their ability and they noted down the coordinates of one character before they drew the picture from someone elses coordinates.
I told the children that if it didn't look right, they should get up and go and ask the person if they were sure they got the coordinates the right way around. The results were really good from this exercise. And because they were drawing characters that they loved they enjoyed it.
Lesson idea 4
Secret messages. Children should be allowed to write out secret messages on a piece of graph paper. They then note down the coordinates for each letter. Making sure that other children knew that a set of coordinates belonged to one letter.
The coordinates are then passed to a different person and they then de-code it.
Lesson idea 5
Writing their names. Children do the same thing as above but this time they write their names and note down the coordinates. They then give this to the teacher. They distribute it to the class who draw out the correct name. They have then found their partner to work with during the day/lesson.
Lesson idea 6
Play battleships. It is very easy to set up a board and get them to draw the ships etc. Some children have never played the game before so be wary of this as you shouldn't be spending much time explaining how to play something which really isn't apart of their learning.
It will need modelling at the beginning but then the children can have a lot of fun playing.
This could be good for little children too if you used a proper board game because it has letters and numbers. So you explain they must use the x-axis first (I can't think if this is the number or letter) then they would recognise the pattern.
Lesson idea 7 (great idea for kinethetic learners)

A brilliant problem solving website
- Home Page : nrich.maths.org
Problem solving activities and descriptions for every piece of maths you want from KS1 to 5.
Lesson idea 8: (Picked up from N-rich)
This activity is a great way to get the children up and moving. The chairs of your class are set out like that of the diagram shown opposite. All facing the same way - towards the front. Every child then sits down on a chair. It does not matter if you have spare chairs as this can be used in your instructions.
Then you need to ask the class a few questions so they know and understand the idea behind this idea:
- If I wanted the person who was in the first row, and the first column to stand up who would that be? (This would be the blue chair)
- Here it would be beneficial to explain to the children that each chair is a point, having a particular position
- Then talk your way through this idea so the children know what it is about.
Once they know then you can speed things up by giving instructions like the following:
- 4,1 and 2,3 stand up and swap places
- Stand up everyone in row 3
- Stand up everyone in column 5
- Stand up everyone who has the same number for the x-axis and y-axis.
This obviously can go on for a long time and get progressively harder.
Extension:
It is possible to introduce all four coordinates into this activity by changing the reference point. Maybe somewhere in the middle could be where the x-axis and y-axis meet. Metre rules or rope could be used to 'draw' this line if the children need this reference.
Once this is explained to the children they can stand up and tell the class their coordinates so they know what it is. Then you can:
- Start off with place-swapping exercise to the one described above.
- Then particular people were asked to stand, for example (1,0) (1,1) (1,2) and (1,3).
They were invited to:
- Say their own coordinates
- Notice what they all had in common i.e. first coordinate of 1
- Observe that they formed a straight line
This led on to the fact that because all the points on that line have an x coordinate of 1, the line is called x = 1.After a few practices of this, we were used to assigning a line its equation and the activities progressed into mirror symmetry. We were told that a particular line was the "mirror line". One point was called, that person stood up and then their reflection in the given line had to stand too. I have to admit that by this stage we had become much slower at jumping to our feet, not simply because of weary muscles but rusty brains too!!
The final challenge was to incorporate rotational symmetry. A point was chosen as the centre of rotation and everyone had to move into their new position after rotation of a given angle in a given direction. This was quite chaotic at first! We then tried another rotation but holding hands with two neighbours. This really emphasised the fact that we had all moved exactly the same amount in the same direction so that our spatial relationships with each other had not changed.
On a practical note, it may be helpful to place (0,0) in the diagonally opposite corner to the blue chair so that pupils have the origin at their left hand side. Depending on the space you have available, you may have to limit numbers and have a group of children at a time.
I came away itching to find a class to try this with! I am convinced that the "hands (or should that be bodies?) on" approach can only enhance our teaching methods. Obviously these activities are progressive so that you could not possibly include everything described in a single lesson, but do have a go and let us know how you get on. If you're feeling brave, why not extend it to 3-d?!!
A brilliant lesson and so much fun for the children - plus it helps relate so much maths together that children will see how they are connected.