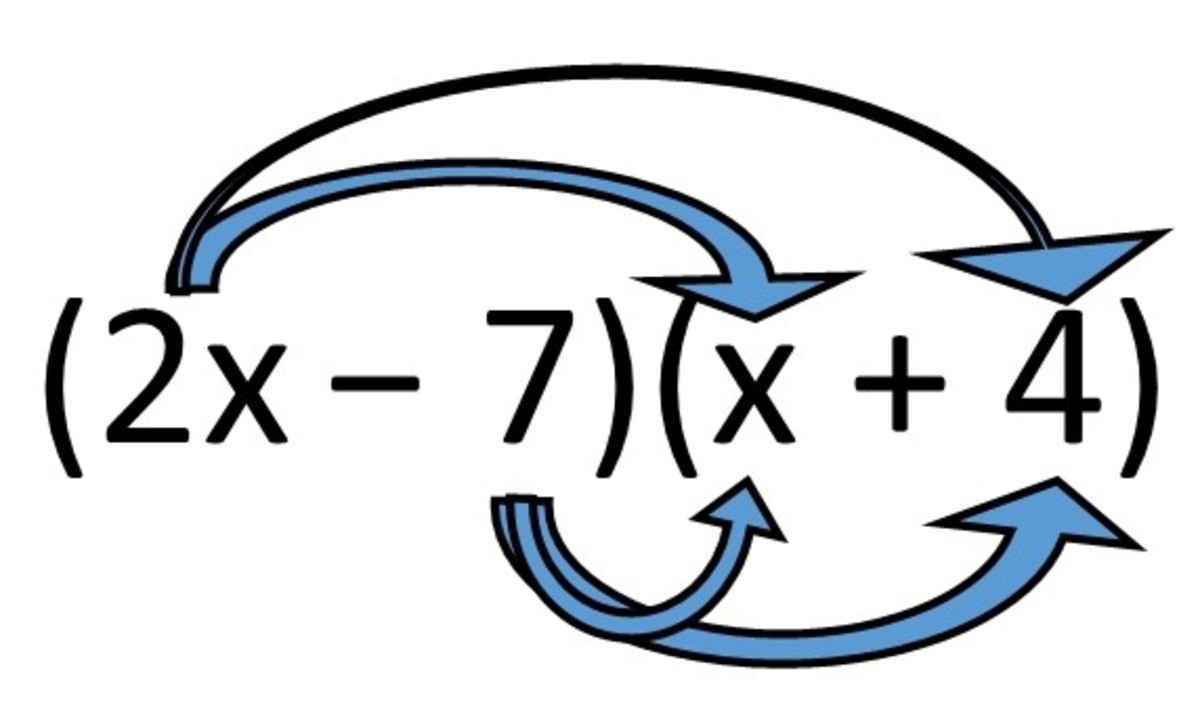Factoring Exponents

There are multiple methods for factoring an expression with exponents.

If you are on this page you are either

1. Trying to factor exponents directly (ex x^{2 + x})
2. Trying to factor expressions with exponents (ex: x²  - 16)

Note: Altough you can factor variables with exponents, you cannot factor the exponents themselves. For example: you can factor the x in (x²+2x), but you cannot factor the 2 in x² + y²

Before explaining how to factor exponents, let's review some terms.

Base: The base is the variable or number in question. 4x²

Exponent: An exponent is the power of a base. It implies how many times that base is multiplied by itself. 4x²

Coefficient: A number or variable that accompanies a base, but isn't an exponent. 4x²

Term: A base (that may or may not be accompanied by a coefficient or exponent). 4x²

Monomial: An expression of a single term. 4x²

Polynomial: Multiple terms that are added or subtracted. 8x³ - 4x² - 2x + 4

Binomial: A polynomial of exactly two terms. 2x + 4

Trionomial: A polynomial or exactly three terms. 4x² - 2x + 4

If you have a single base whose exponent happens to be an expression, you can indeed start factoring exponents. If the terms are separated by a +, you make another factor with the same base and just move over the term that had been separated by +. If the term was sepatated by a - sign, you do the same but instead of multiplication, you use division.

Example:

• x^{2y + 2} becomes (x^2y) (x²)
• x ^y-2 becomes (x^y) / (x²)
• 3x^{9x^2 - 16} becomes (3x^{9x^2)} / (3x¹⁶)
• (The above example can also become 3x^(3x-4)(3x+4) if you use the difference of squares method mentioned below, though this probably won't be very useful. I just want to point out that the rules for factoring polynomials also apply if the polynomial is within an exponent - should you choose to do so)

One way to factor is to see what two sets of terms share

Example:

• Given the expression: 2x² + 2y
• 2x² are factors 2 * x * x
• 2y are factors 2 * y
• They have 2 in common

So what do I do with that?

You take the common factor(s) aside. You then put the original terms in a parenthesis and divide the common factors from each term. Put the parenthesis and the set-aside factors together.Example:

• We established that 2x² and 2y have 2 in common
• 2 is set aside
• (2x² + 2y) divided by 2 becomes (x² + y). Set aside.
• Put both back together to make 2 (x² + y)

NOTE: The common factors don't have to be numbers! Variables can be common factors too! so you can factor them out too. When you take out a common variable, you reduce the original exponent by 1.

Examples:

• 3x³ + 2x both have x in common. They become x ( 3x² + 2)
• x³ + x² both have x in common as well. However, notice how the exponents go to 3 and 2? That means your variable in common goes up to 2. So in reality, x² is their common factor. This factors into x² (x + 1)

If you have a polynomial where both terms are squares and one of them is accompanied by a negative/minus sign, you can use difference of two squares. This can be used for factoring exponents that are even.

Examples:

• x² - 9 is (x)² - (3)²
• -4x² + 1 can be rewritten as 1 - 4x², which is therefore (1)² - (2x)²

Ok, but what do I do with it?

You can make it into two factors by using the numbers within the () above and putting them as two terms. In one factor these terms are separated by -, and in the other, they are separated by +.

Examples:

• We already established that -4x² + 1 can be rewritten (1)² - (2x)^2. Therefore it factors into (1 - 2x) (1 + 2x)
• We also established that x² - 9 is (x)² - (3)^2. This factors into (x - 3) (x + 3)
• 9x² - 16 is (3x)² - (4)². Therefore it factors into (3x - 4) (3x + 4)

Trinomials of power 2 can be factored using their own special way.

Trinomials of power 2??

Yes, trinomials where the highest exponent is 2. Generally trinomials that look like ax² + bx + c.

Examples

• x² + 4x + 2
• y² + 7y + 6
• x² - x - 6
• 2x² + 9x + 7 (polynomials like this would need the eyeglass method in order to yield the answer of (x + 1)(2x + 7) )

Going over Trinomial factorization, as well as the eyeglass method, would take far too long for this one hub so here is a video to help you get going!

Factoring a polynomial of degree bigger than 3 can be difficult, unless you're lucky enough to factor out some of that degree. (example x^3 + x^2 + x can be factored into x ( x^2 + x^1 +1) and therefore both of the factors would be in degrees less than 3. So further factoring using the basic techniques mentioned above would work)

If you aren't so lucky, chances are you won't be able to factor using regular techniques. You will need to do either factor or synthetic division (both of which allow you to divide out binomial factors) by taking guesses on what you think one of the factors is. Using a graphing calculator might help you find the zeroes of the equation, which in turn can be used to help you do either factor or synthetic division.