Basic Fractions (Curious Concepts Precalculus 1.4)

Self-explain Learning Technique
Self-explain Learning Technique helps people to better comprehend, and remember any thing they want to learn. It my sound strange, but it is many times more effective then re-reading. To use this technique, give short answers to the prompted questions. The more previous knowledge-and-life experience that you incorporate into your answer, the more effective this learning technique is. On average it improves learning by over 2.5 standard deviations; the difference between a C student and an A student.
How have you struggled with fractions?
Fractions are the way we express parts or groups of parts: 2/3 means that we have 2 of 3 parts. This can also be conintued on past a complete whole: 4/3 means we have 4 of 3 part; we have enough 1/3's to make 1 complete whole and have another one 1/3.
Strangely, it is more to know how to muliply and divide fractions before we try to add and subtract them.
Multiplying Fractions
We multiply fractions by multiplying the numerators together and place it over the product of the denominators. This can easily be remembered as multiplying straight across.
(a/b)(C/D) = (aC)/(bD)
Example:
(3/7)(6/5) = [3(6)] / [7(5)] = 18/35
Self-explain Learning Technique
How would accidentally flipping the number being divided effect your answer?
Dividing Fractions
Dividing fractions is very similar to multiplying fractions. The key difference is that we flip the fraction that we are dividing by before we multiply straight across.
(a/b) / (C/D) = (a/b)(D/C) = (aD) / (bC)
Adding Fractions
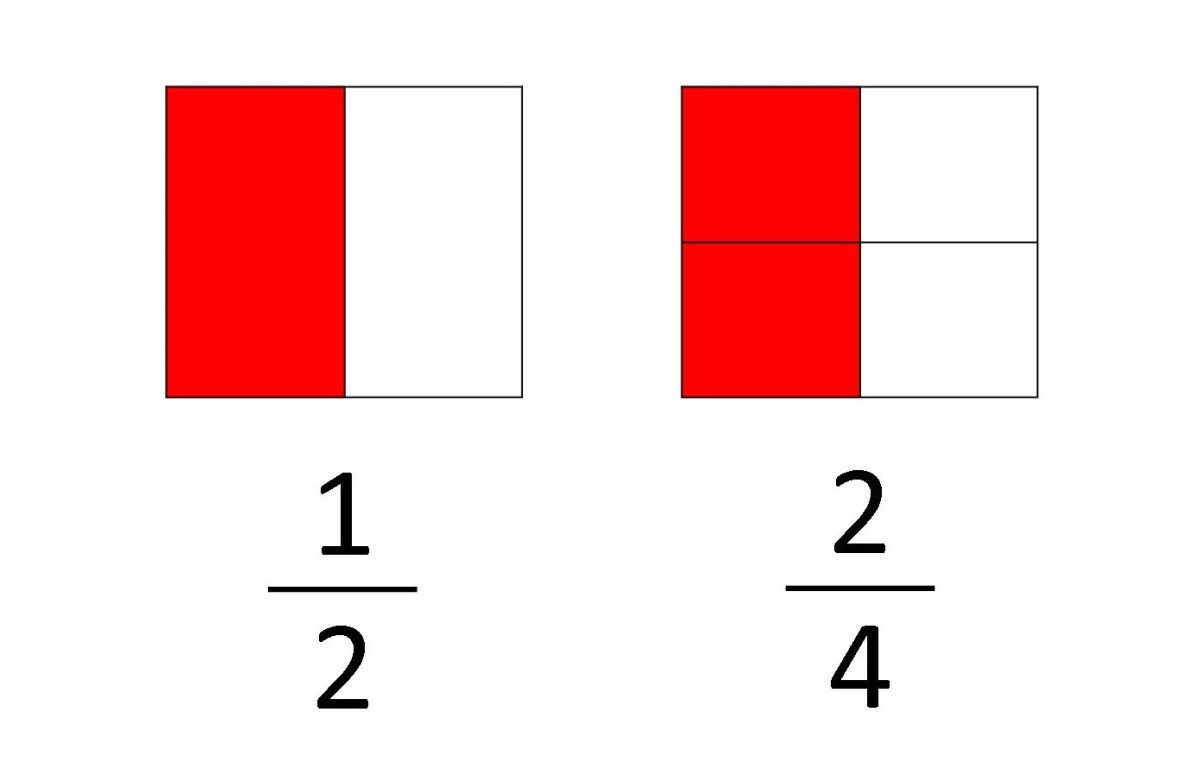
We always try to express a fraction in the easiest way to understand it. We can do this by making the bottom number the smallest possible Integer. If we have 2/4 we express it as ½. We know that 2/4 is the same as ½ because both 2 and 4 can be divided by 2. This will reduce 2/4 into ½, making it easier to understand.
What if we wanted to add 7/3 to ½? We first start by finding a common denominator. The simplest and easiest way to get a common denominator is to multiply both fractions by 1 ;). 1 can be expressed as 3/3 and 2/2. We multiply 7/3 by 2/2, getting 14/6. Then we multiply ½ by 3/3 getting 3/6. now we can add: 14/6 + 3/6 = 17/6. We only add the top number (numerator), the bottom number (denominator) remains the same.
We can not reduce 17/6 because 17 is not divisible by 3 or 2. We can leave it as an improper fraction almost anywhere other then basic math courses.
If we are in a basic math course, we want to better understand the value of fractions greater then 1. This is why we do not use improper fractions as our final answer. If we are given the fraction 17/6 we can turn it into a mixed number. First we find out how many times 6 goes into 17. 6 goes into 17, 2 times. We write 2 as a whole number. Next too the 2 we write the remaining fraction: 5/6. our final answer will be 2 and 5/6.
Self-explain Learning Technique
How would you add to a negative fraction?
Subtracting Fractions
Subtraction is the same as adding a negative: a-b = a+(-b). We subtract fractions just like how we add them. The only minor difference is that we are adding a negative value: 5/2 + (-1/2). Since the denominators are the same we just subtract the top numbers or add a loss: 5-1 = 4. We place this over the denominator and get 4/2. The Numerator, 4 can be evenly divided by the Denominator, 2. Our final answer will be 2, because 4 halves is the same as 2.