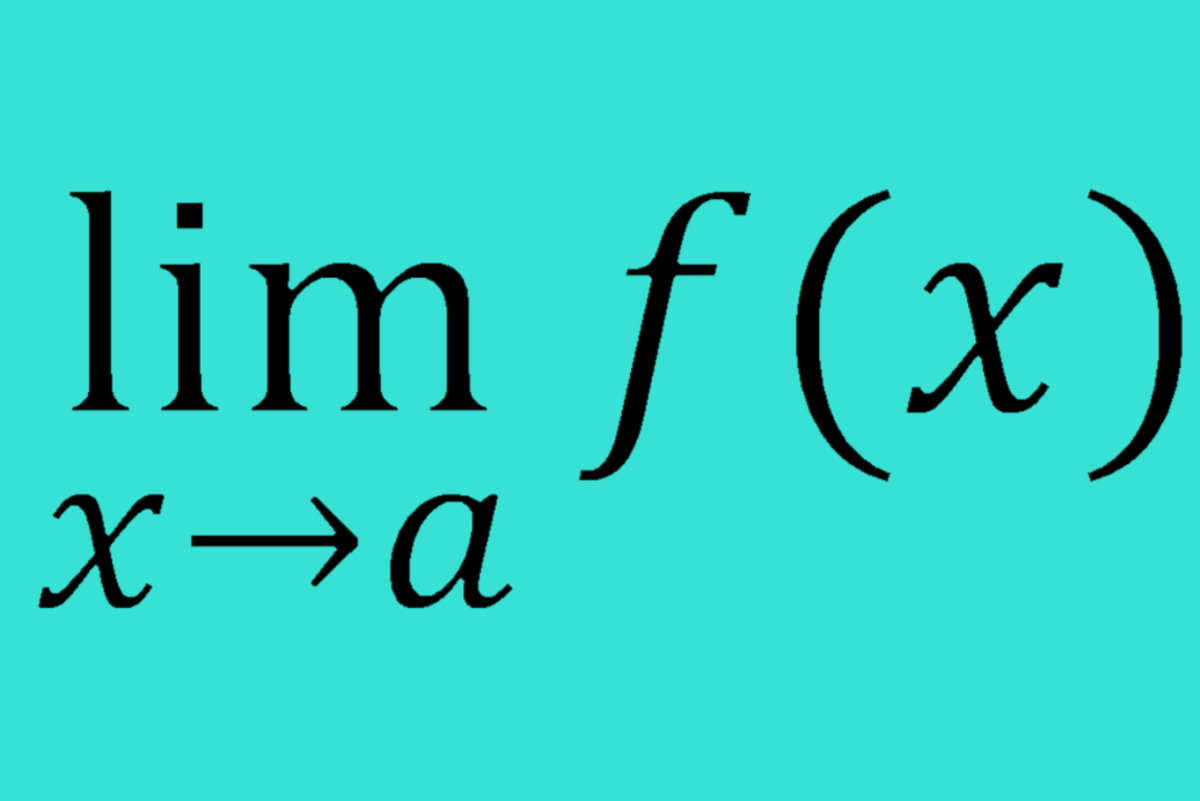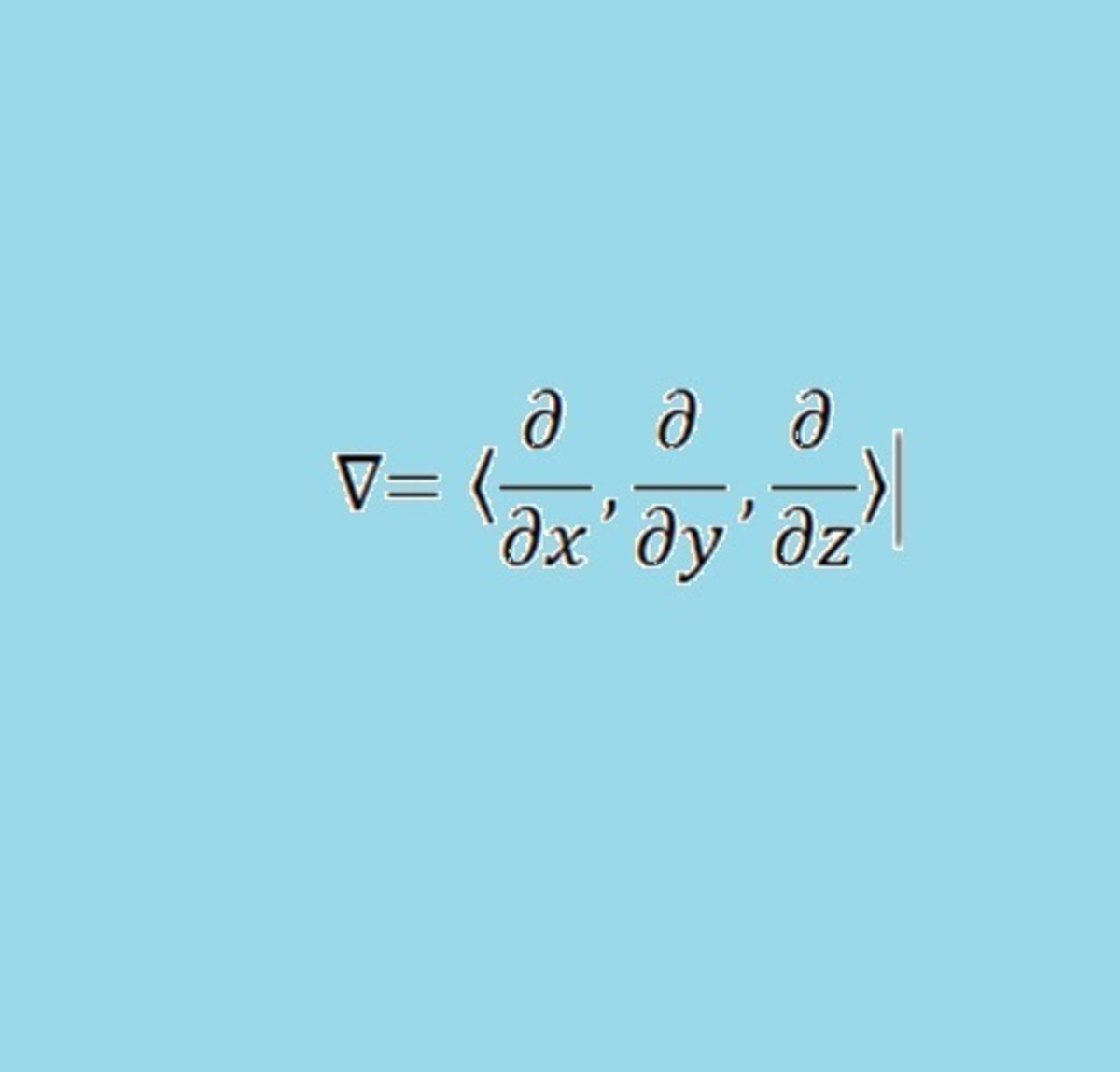Introduction to Calculus: Studying limits

This hub is just a basic introduction to limits. Hopefully, if time permits, I will be writing about limits involving absolute value, trigonmetric functions and eventually the 'derivative' limit in another hub.

Let's first define what a limit is: The basic definition of a limit is the value the y as x or the independent variable approaches a certain value.

Let us start with a quick example.  lim_x→5(2x-5)

The value of where the function is approaching should be directly under the lim.  The question is asking for what the value of the closest possible value of the function when x approaches 5.  Please realize that I stated that it is the closest possible value.  In some cases, there may be a hole, but the limit would still exist.  But in a case like this, there should not be a problem.  There are two limits (one from the left and one from the right); again, in this case it should not make much of a difference.  So let's begin.  The easiest way to go about this problem is to plug in the value of x (which is 5). 

2(5)-5=10-5=5

The limit is 5 because that would have been the closest y or f(x) value when x approaches infinitely close to x=5.

Let us try one more 'nice' example.

lim_x→3(x^3-2x+5) = (3^3-2(3)+5)=9-6+5=3+5=8

Now let us try an example that would lead us into a trap.

Given the functions:

y=5x-3 x>5

y=x when x<5

find the limit of this graph when it approaches 5.

Now there is no value at five for each of the functions and the functions approach different numbers as they both approach x=5!  The answer for this case would actually be there is no limit because IF THERE ARE TWO DIFFERENT LIMITS FOR A GIVEN X, THEN THE LIMIT DOES NOT EXIST IF THERE IS NO CONDITION ON THE LIMIT.  Now this brings me to my next point of discussion.  If the question asked for the limit of x=5 as it approached the left (designated as lim_x→5^- ...the minus sign means 'from the left'), then we must find the function that came from the left of 5.  What designates all numbers less than 5?  x<5.  So what is that function? y=x.  So all we have to do is plug in 5 into x (which is 5) and that is our limit.  If it asked for the limit as x approached 5 from the right (lim_x→5⁺ ), then we would use the 5x-3 function.  Again, even though the function at x=5 for both the functions do not exist, the limit is not asking for the value at the point, but the closest value approaching the limit.