Can You Solve Larry's Polygon Puzzle?

Background
When we studied plane geometry in high school, we learned the concept of congruent polygons. Basically, two plywood polygons are congruent if we can put one of them on top of the other one, and if all of the sides are flush with each other. It may be necessary to flip one of the two polygons in order to achieve congruence, even though flipping is not explicitly stated in Euclid's postulates.
We also learned several congruence theorems for triangles. Here are two examples:
Two triangles are congruent if two of the sides--plus the angle between them--of Triangle A match up with the corresponding ones of Triangle B. Ditto for two angles and the included side.
These are known as Side Angle Side and Angle Side Angle, and by the acronym, SAS and ASA, respectively. Other congruence theorems include: SSS (Side Side Side) and AAS (Angle Angle Side).
However larger polygons--quadrilaterals, pentagons, hexagons, heptagons, octagons, nonagons, decagons, etc.--are trickier. And the friendly congruence theorems that work so nicely for triangles do not necessarily apply to more complicated polygons.


The puzzle
An N-gon is a polygon with N sides and N angles. Suppose that we have two plywood N-gons. Let's also assume that there's a one-to-one correspondence between the sides of N-gon A and those of N-gon B. However the matching sides of the two n-gons are not necessarily in the same order.
Let's make a similar assumption about the angles of the two N-gons. Let's also assume that N-gons A and B are not triangles. Then it is not necessarily true that N-gons A and B are congruent.
The puzzle: What is the smallest possible value for N, such that N-gons A and B are not necessarily congruent? And yes, flipping is allowed.
In other words, we're looking for two NON-identical N-gons whose sides and angles match up, one for one, but in different orders. Having found one such pair of N-gons, can you find another pair of 'paradoxical' N-gons that have a smaller value of N?
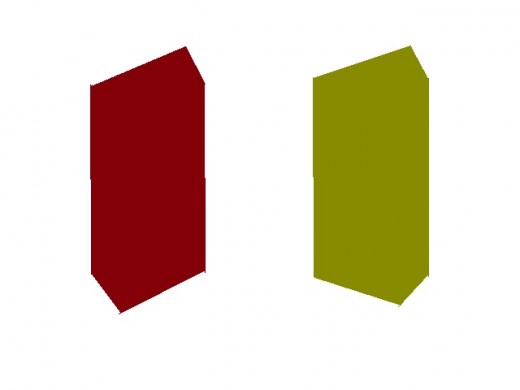
A hint and a few more things
This puzzle illustrates the idea that muddling, which has gotten a bad reputation, is a perfectly valid problem-solving technique.
Having a mathematical background will not be particularly helpful in solving this puzzle. Formulas and equations are not relevant here. The only tools that you need are a pencil, a ruler, a protractor, and lots of paper. Or you can treat it as a 'pillow problem'.
Hint: The two non-identical hexagons in these crude hand drawings have identical sides and angles. However their orders are different. Pentagons, anyone?
About Racetrack Playa
I chose the photo at the top of this hub, because it has lots of small polygons in the foreground. Racetrack Playa is arguably the biggest mystery of Death Valley National Park. We're still not sure about how these large stones move around, leaving tracks in their wake.
Copyright 2011 and 2015 by Larry Fields