Larry's Tetrahedron Puzzle
What is a tetrahedron?
A tetrahedron is basically a pyramid with a triangular base. It has six edges, four sides, and four corners. "Tetra" means four. See the figure at right.
In a Regular Tetrahedron, all of the edges have the same length. A Regular Tetrahedron qualifies as a Platonic Solid, because all of the faces are equilateral triangles, and the same number of triangles meet at each corner.
A Regular Octahedron is also a Platonic Solid. This eight-sided solid can be made by gluing the bases of two square pyramids together.
A Regular Icosahedron is also a Platonic Solid . This beautiful shape has 20 equilateral triangles as faces. Five of these triangles come together at each corner.
An Australian reader points out that a Regular Tetrahedron is the most difficult Platonic Solid to tip over. For this reason, Regular Tetrahedra have been used as barriers to tanks in war zones.
Regular Tetrahedra are of particular interest to chemists. A few simple molecules have tetrahedral shapes.
One such molecule is methane, whose formula is CH4. A methane molecule has one central carbon atom, and four hydrogen atoms. The latter are located at the corners of an imaginary Regular Tetrahedron.
Speaking of tetrahedra, there's even an Organic Chemistry journal, called Tetrahedron.
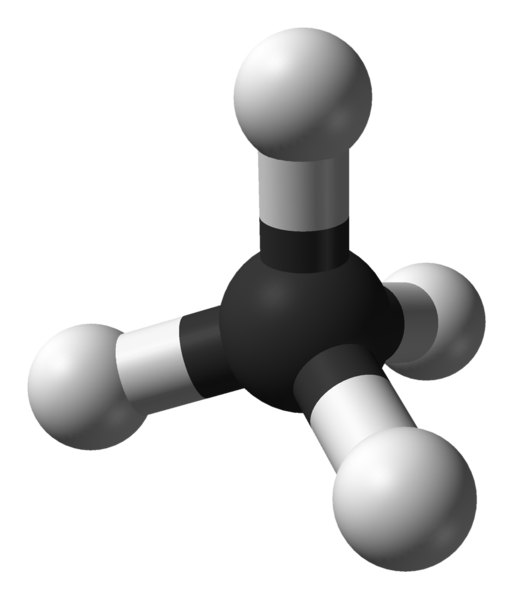
Methane
Methane is the principle component of natural gas. Because pure methane is odorless to humans, a little stinky stuff is added, so that people can smell natural gas leaks in their homes, and take the appropriate safety measures.
Methane has been in the news in recent years. Because of a hydraulic technique called fracking, methane can be extracted from deeply buried shale rock.
Estimates of world-wide natural gas reserves are much greater than they were 10 years ago.
As is the case with many technologies, there are environmental questions regarding fracking. After we learn more, additional government regulations may be prudent.
Methane plays an important role in the generation of electricity. Electric utilities must plan for two types of power generation: Base Load and Peak Load. Coal-fired power plants are reliable and cheap for generating Base Load power. However they cannot be ramped up quickly. Ditto for nuclear power plants.
This is where natural gas turbines come into the picture. These are very dispatchable for Peak Load electrical requirements.
The puzzle
A Regular Tetrahedron is 1 meter high. What is the distance from the base to the center?
Yes, one could solve the problem, using trigonometry, together with iterations of the Pythagorean Theorem. But that brute force approach would not be very sporting, and it would be a bit messy. Fortunately, there's a simpler way to solve the problem at hand.

My solution
One can construct a Regular Tetrahedron, by gluing together four shorter tetrahedra having the right height. Each short tetrahedron has the same base as the Regular Tetrahedron.
Thus the Regular Tetrahedron has 4 times the volume of a short tetrahedron, and four times the height, since the volume is proportional to the height.
The height of a short tetrahedron is equal to the distance from the the base to the center of the larger, Regular Tetrahedron. And that distance is 1/4 meter. Voilà!

The upshot
Have you ever wondered about the "3" in the magic formula for the volume of ordinary square-base pyramids (and for tetrahedra)?
V = bh/3
where V is the volume, b is the area of the base, and h is the height.
Why do we divide by 3? No, the formula did not appear on a stone tablet given to benighted humans by benevolent, all-wise Geometry Gods from Mount Olympus. There's a good reason for it.
Hint: One can apply a logical process similar to that used in solving the Tetrahedron Puzzle. Start by gluing together six short, square-based pyramids to build a cube.
Here's an interesting coincidence: Once we know how to find the volume of a pyramid, the same formula will yield the volume of a cone. Or is it a coincidence?
We have the Cavalieri Principle to thank for the versatility of this particular volume formula. Suppose that two objects sitting on a table have the same height. Suppose further that the cross-sectional areas of the two objects at every given height are always equal. Then the two volumes are also equal. It's elementary, my dear Watson.
The volume formula for objects with flat bases and pointy tops is a geometric example of the BOGOF Principle. This comes from the advertising slogan: Buy One, Get One Free. By the way, I used the BOGOF Principle to prove Larry's Theorem in a mathematical hub about the First Digit Problem.
Copyright 2012 and 2013 by Larry Fields