Eudoxus
Eudoxus was born in Cnidus (or Knidos), an ancient Greek city in Anatolia, now part of Turkey in around 410 BC. Eudoxus spent some time at Tarentum (Taranto) in southern Italy where he studied mathematics under Archytas, before moving on to Athens where he studied under Plato at The Platonic Academy. As well as mathematics, he also studied medicine and astronomy.
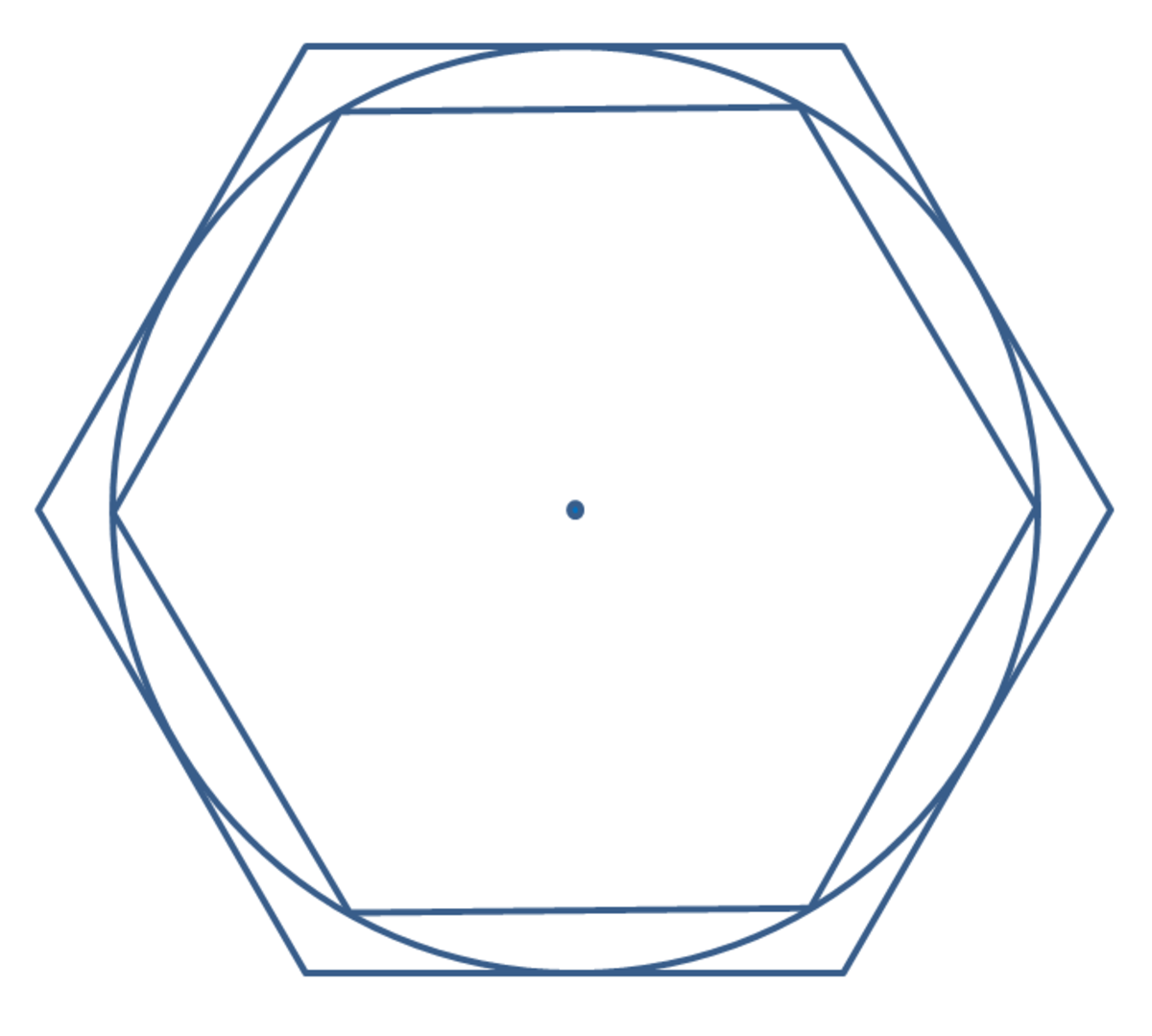
Eudoxus is famous for his method of exhaustion which he applied to find the perimeters, areas and volumes of shapes that do not have straight edges. An example of Eudoxus’ method is to find the circumference of a circle by inscribing and circumscribing the circle with regular polygons. Then by increasing indefinitely the number of sides of each of these polygons, he was able to find two values between which the circumference of the circle lay. The more sides each of the polygons has, the more close their perimeters will be to the circumference of the circle. Essentially Eudoxus used what we would today call a limit. He applied this method to other curves, and also to finding areas and volumes of shapes with curved edges.
Eudoxus is also famous for his definition of equal ratios (see below).
Eudoxus’ other claim to fame is in astronomy for his ingenious model of the solar system. In an attempt to model the observed belief that the moon and the sun rotate around the earth, Eudoxus gave to each of them three spheres. The three spheres for the moon respectively explained its rising and setting, its monthly motion through the zodiac and its monthly revolution. The three spheres for the sun were similar except that its motion was annual rather than monthly.
Eudoxus spent his final years in Cyzicus, northern Turkey, where he taught mathematics and practised medicine, before returning to his native Cnidus. He died in around 355 BC.
A contribution of Eudoxus to mathematics
One of Eudoxus’ great contributions to mathematics is his definition of equal ratios. The early Greek mathematicians, influenced by The Pythagoreans, had a real problem with irrational numbers since their philosophy was based on the assumption that all mathematics is constructed around the whole numbers. It was considered impossible, therefore, to compare incommensurables such as the length of the side of a square and the length of its diagonal. Today, we have no problem in expressing this ratio as 1:Ö2, but the early Greeks could not accept a ratio between rational and irrational numbers.
This definition of Eudoxus’ equal ratios is given by Euclid as Definition 5 of Book V of ‘The Elements’. This translation is taken from ‘Men of Mathematics’ by ET Bell (Pelican books, 1965):
The first of four magnitudes is said to have the same ratio to the second that the third has to the fourth when, any whatever equimultiples of the first and third being taken, and any other equimultiples of the second and fourth, the multiple of the first is greater than, equal to, or less than the multiple of the second, according as the multiple of the third is greater than, equal to, or less than the multiple of the fourth.
This is what it means in modern-day language:
Take four magnitudes a, b, c and d, with a and b being of the same kind (e.g. both lengths of line segments, both areas, both volumes, or both angles) and likewise c and d are of the same kind, and such that the ratios a/b and c/d are equal. Then, if m and n are arbitrary positive integers, one of the following is true:
If ma > nb , then it must also be true that mc > nd
If ma = nb, then it must also be true that mc = nd
If ma < nb, then it must also be true that mc < nd
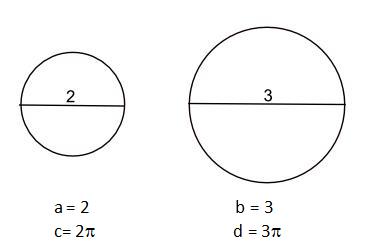
Take, for example two circles with diameters 2 and 3.
If a and b are the lengths of the diameters and c and d are the lengths of the circumferences, then we know that a = 2, b = 3, c = 2π and d = 3π
Choose arbitrary positive integers m = 5 and n = 3, then:
ma = 5 × 2 = 10
nb = 3 × 3 = 9
mc = 5 × 2π= 10π
nd = 3 × 3π = 9π
So it is true that ma > nb implies that mc > nd
Note that we have compared the magnitudes, and that the units were not specified.