Democritus
The beginnings of the integral calculus do not belong with the great 17th century mathematicians, Newton and Leibniz, or with their immediate precursors such as Cavalieri, nor even to ‘The Method’ of Archimedes. They can be traced back to a little known Greek mathematician named Democritus.
Democritus was born in about 460 BC in the town of Abdera, Thrace, in the northern part of Greece. His Abderic school of thought believed in atomism. They believed that everything, even the mind and the soul, is made up of atoms that move around in a void. These atoms are particles that come in various shapes and sizes, but are indivisible. His idea for the physical world is not very different from the modern concept of the atom.
According to Archimedes, Democritus applied the idea of atomism to geometry by using infinitesimals to find the formula for the volume of a cone. He was the first mathematician to give the formula for the volume of a pyramid – not just a square-based pyramid - but one whose base is a regular polygon with any number of sides, including the cone (which is just a circular-based pyramid).
The formula for the volume of a square-based pyramid was known to the Egyptians and Democritus may well have learned of it during his travels. But he expanded on this by giving the formula
Volume = 1/3 × Area of base × Height
for any pyramid. The fact that he inferred the formula for the volume of a cone by regarding its base as a regular polygon with an infinite number of sides of infinitesimal length is the first example of a technique that forms the basis of the integral calculus.
A contribution of Democritus to mathematics
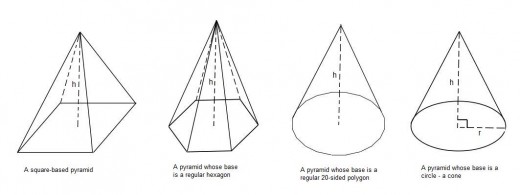
Democritus showed that the volume of each of the first three pyramids is given by the formula
Volume = 1/3 × Area of base × Height
In the case of the pyramid whose base is a regular 20-sided polygon, for obvious reasons, I have not drawn in all the sloping edges from the vertex to each of the 20 points around the base. It is clear, however, that there are 20 straight edges around the base and that the polygon is very close to being a circle. Imagine a similar pyramid whose base is a 100-sided regular polygon – it would be almost indistinguishable from a circle.
Democritus used his atomist ideas to show that, as the number of sides of the polygon increases, the polygon gets closer and closer to being a circle, and becomes a circle when the number of sides is infinite. In modern terms we would say that ‘in the limit, as the number of sides of the polygon tends to infinity, the polygon tends to a circle’.
Of course, in the case of the circular based pyramid – the cone – the formula becomes
Volume = 1/3 × πr2 × h where r is the radius of the base.