Jump: Physics of Ski Jumping

Ski Jumping is a sport in which competitors ski down a steep ramp or ‘In run’ with a take off ramp which they use to jump into the air. Ski jumping originated in 1809 in Norway, with the first completion held in 1862, and has been a part of the Winter-Olympics since the first games in 1924.
The jump is scored on a system based on distance and style. Each ‘in run’ has a par distance to aim for (the K-Point), for example at 90m from the ramp. Skiers are awarded 60points for landing on the K-point or lose 1.8 points for every meter they are away. 5 judges can also award up to 20points for the style: focusing on keeping the skis steady, balance, body position and landing.
Physics ofSki Jumping
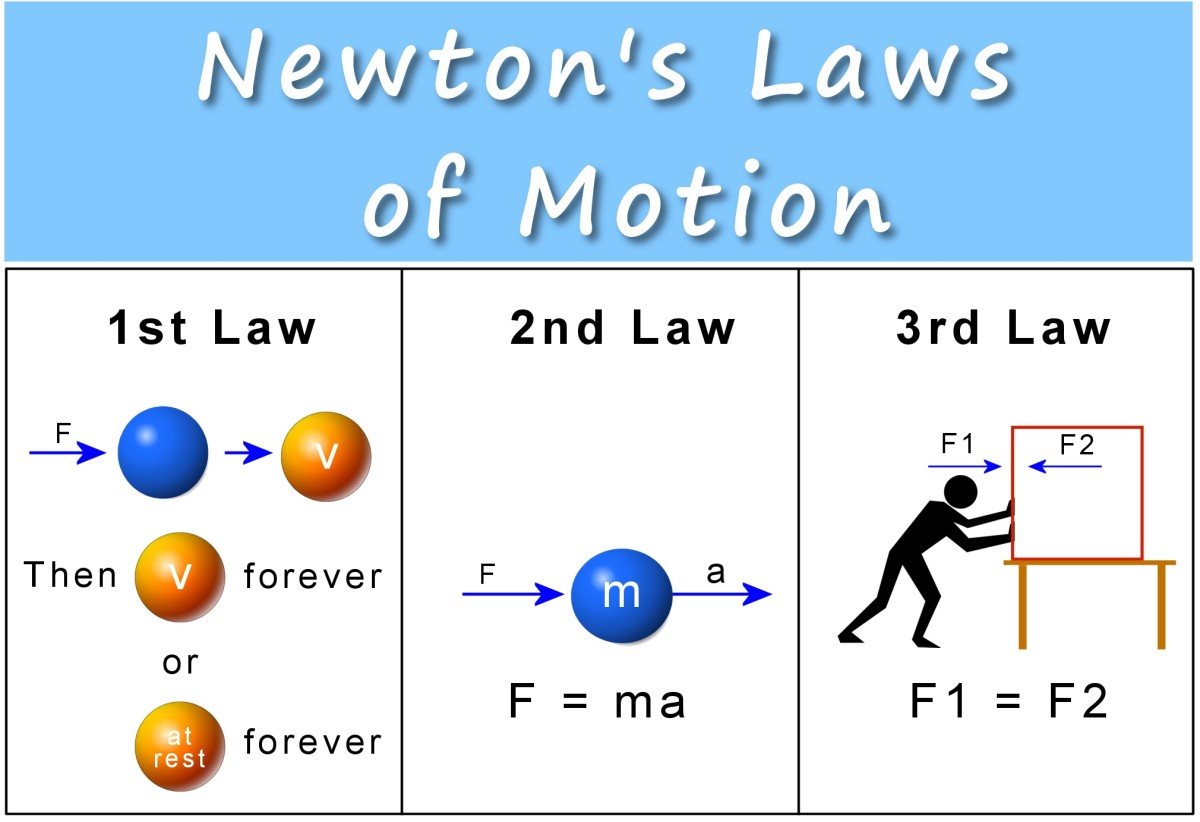
As in normal skiing the run down the ramp is based on a basic energy transformation from gravity potential energy to kinetic energy.
Kinetic Energy: Ek = 1/2mv2
Gravitational Potential Energy: Egrav = mgh
Other Top Physics Hubs:
Therefore, the kinetic energy a ski jumper possesses is based on their velocity on take off and this is based on their initial gravitational potential energy. The conversion from gravitational potential to kinetic energy is not 100% efficient as energy is lost through friction, as sound, drag and heat. Ski jumpers attempt to optimize their jump by reducing drag; they do this by leaning forward and keeping their skis parallel to their direction of travel. This minimizes the area of their body pushing through the air and streamlines them, therefore reduces drag. Also, due to being more streamlined, the amount of turbulent flow around them in flight is also decreased, maximizing their speed.
By angling their skis into a ‘V’ shape ski jumpers can maximize the lift generated by increasing the surface area of air flowing over their skis, generating more lift, helping them stay in the air longer.
The principles of projectiles can be applied to a skier’s trajectory. When a skier leaves the ramp his direction of travel can be resolved in two directions, horizontally and vertically. If they were to leave the ramp at an angle Ѳ then the velocity in the vertical component can be given with:
Velocity x sinѲ - This will affect how long the skier remains in the air and will be affected by vertical drag and the skiers weight force pulling them downwards.
The velocity in the horizontal component can be given with:
Velocity x cosѲ - This will affect how far the skier travels and is affected by drag and any lift generated during flight.