Velocities in Special Relativity
Relative Velocities at Low Speeds - Easy!
Imagine a situation in which two cars are on a direct collision course towards each other, both travelling at 50 mph. What is the velocity of the cars relative to each other? I.e. if you were sitting in the first car, how fast would the second car be speeding towards you? Usually this is an easy question to answer: the relative velocity is the second car's velocity minus the first car's velocity. Relative velocity = 50 mph - (-50 mph) = 100 mph
Relative Velocities at High Speeds - Not So Easy...
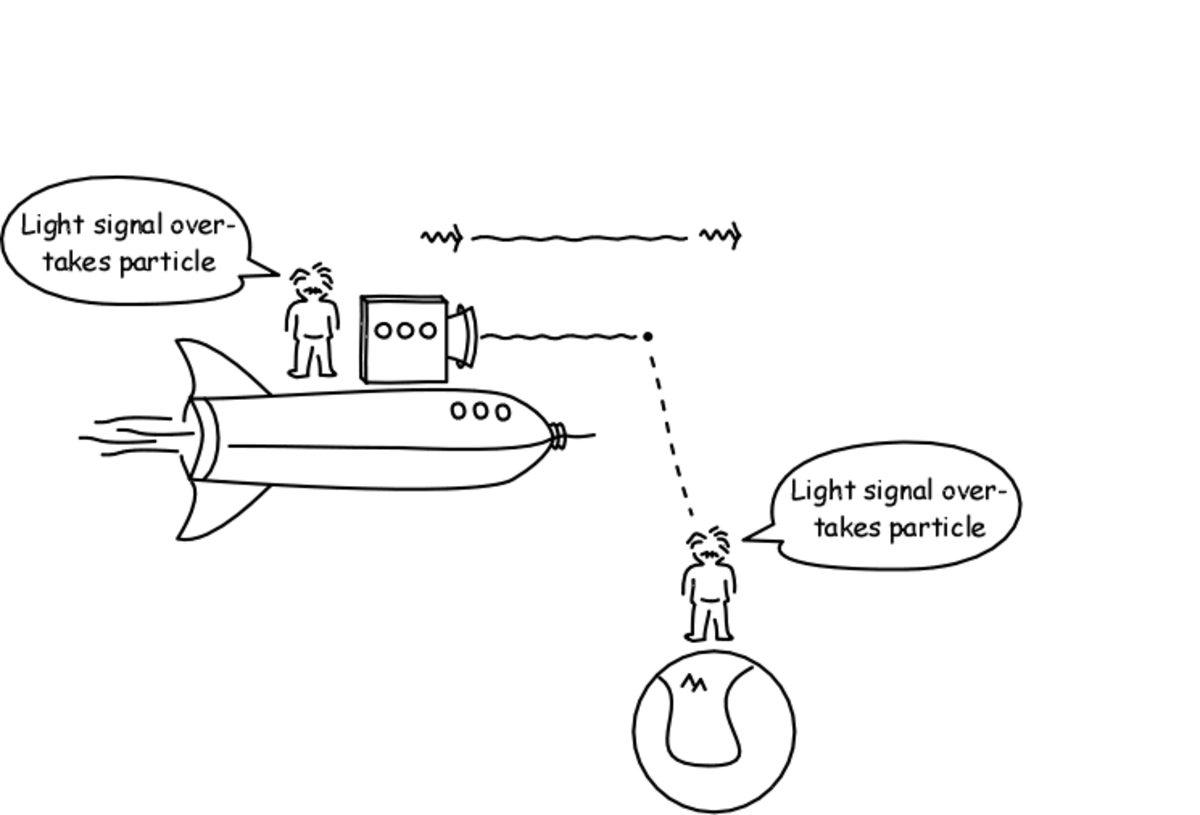
However, when the vehicles are travelling at close to light-speed (186282 miles per second), it's not so straightforward. This is because of Einstein's theory of special relativity, which says that all observers, no matter how fast they are travelling, must observe the speed of light to be 186282 miles per second. No matter how fast you run after a beam of light, it still appears to be travelling away from you at 186282 miles per second.
This is only possible if the definitions of "miles" and "seconds" change. What looks like a mile to the person travelling at high speed actually looks like much less to a person who is standing still. This is called length contraction. Similarly, what feels like a second to the person travelling at high speed actually feels like much more to a person who is standing still. This is called time dilation.
Velocity Composition Formula
In order to take account of this, you need to apply a correction factor to the calculation of relative velocity.
Instead of the simple formula that applies at low speeds:
Relative Velocity = (v1 - v2) (where v1 and v2 are the velocities of the first and second objects)
In special relativity, we need to use the formula:
Relative Velocity = Γ (v1-v2)
where the correction factor Γ = 1/(1+v1*v2/c^2) and c is the speed of light.
Example Calculation
A ------------> <-------------B
Consider two particles, A and B, on a collision course. Each is travelling at 75% of the speed of light. Calculate their relative velocity as follows:
v1 = 0.75 c, v2 = -0.75 c
Relative velocity = (0.75c - (-0.75c) ) / (1+ 0.75^2 c^2/c^2) = 1.5 c / (1+0.75^2) = 0.96 c
Note that if you used the non-relativistic formula, you would get the incorrect answer 1.5 c - this is clearly wrong because nothing can travel faster than the speed of light!
topquark works as a researcher in theoretical particle physics and blogs about research at The Particle Pen.