You used to used a slide What?

In a day when scientific calculators can be bought for under $10 and when a $150 graphing calculator Can do math problems better than a computer which took up an entire room in the 1960s, the idea of using a tool as primitive as a slide rule seems ridiculous. However, at the risk of showing my age, I'll let you know that as a requirement to starting classes my freshman year of college I was required to buy a $50 piece of bamboo which was the only tool I was allowed to use in my calculus class. I'm not saying that calculators didn't exist, I'm just saying they were nowhere near as useful as they are today. For that reason, they were not considered a valid tool. Now I haven't done a survey on this, but I'd be willing to bet that there are very few people today who are competent at using a slide rule.

Now, to understand how a slide rule was put together, you need to understand another mathematical term, which is in use today. This term would be a logarithm. Now for some reason, students get all confused by logarithms. However, the logarithm is nothing but an exponent that you put a base to, to get a number. For example, in 10 squared is 100, 10 is the base, two is the exponent, and 100 is the answer. We therefore could say that the log of 100 is 2.

Now there is a property of logarithms that makes them useful in scales. And that is that when you have something which is growing exponentially that goes right off the graph quickly, you can make it linear, meaning a straight line graph, by simply graphing the logs of the numbers instead of the numbers themselves. This property is one of the properties which make slide rules work.

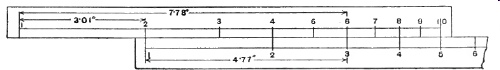
You also have to understand that every number has a logarithm. For example, the log of three is approximately .477, and the log of 30 is 1.477, and the log of 300 is 2.477, and the log of 3000 is 3.477, and so on. This is based on the fact that when you are multiplying numbers having the same base with exponents you add the exponents. This is what made logarithms so popular with mathematicians and scientists of yesteryear, they turned somewhat complex multiplication and division problems into relatively simple addition and subtraction problems.

Now if you take two rulers and you want to add 2+3, you could put the second ruler with its beginning at two and go up to three on the second ruler and you'll notice that on the first ruler that point is five. This is the basic for the slide rule. If I wanted to take 7-3 I could take the second ruler and put the three on seven of the first ruler and go back to the beginning of the second ruler and it will be at the number four. Now in using exponents, you add them when you want to multiply, and subtract them when you want to divide. So all a slide rule is, is two rulers with logarithms instead of inches as their scale. Now on an actual slide rule, there are several scales, including one just like the ruler which is used for addition and subtraction problems. But no matter which scale used, they all essentially work the same way.

Well technically it's not important, just like what kind of pottery a Neanderthal used doesn't really affect your day-to-day existence. It is historical, and probably the only it time would  become 100% absolutely vital, would be in the case of some catastrophic event, where we would lose all electronic devices, and for some off the wall reason some engineer absolutely needed to build a bridge that would support a brigade of elephants. Since this is not likely, I'll just say some people might find it interesting.

Trust me I would not give up my TI-89 for a great slide rule at this point, but I might buy a slide rule for the sake of nostalgia. (by the way, I wonder what that $50 slide rule would be worth today.) This is similar to the reasoning used by auto buffs for buying an old model T. Ford, the main difference being, that restored, that model T. Ford would be worth a heck of a lot more than a slide rule.

So in closing, I'd like to say that we have come a long way in the last 50 years. The days of trying to look smart by carrying around a slide rule and wearing a pocket protector are long gone, in fact if we saw someone walking around today carrying a slide rule we might just think that something was wrong with him mentally. For that reason, if I do go out and buy a slide rule, I'll be sure not to carry it around with me.

Ron is semi-retired and runs The Math Doctor Tutoring Service in Glendale, CA http://www.the-math-doctor.com as well as an environmental website.