How to Subtract Fractions in 5 Easy Steps

The subtraction of fractions is not as difficult as it seems. The key to subtracting fractions is to make them compatible. For example before you can begin to subtract 1/3 from 1/2 you have to convert the 2 fractions so that they have the same denominator (if you don't know what a denominator is, check the definitions, below).

Sometimes a common denominator just jumps out at you, but if not just multiplying the two denominators will always result in a common denominator. Once you have mastered the technique for finding a common denominator and converting fractions so they are compatible, you will be able tackle the subtraction (and addition - which uses the same technique) of fractions with confidence.

This article will walk you through some examples and also includes a video demonstration. For best results, I would recommend reading this article and then watching the video (see link to YouTube video, below).

There are a few terms that are regularly used and you need to be familiar with them:

Mixed Number = 2¼ - where a whole number is used with a fraction.

Improper number = 9/4 - this is where the numerator (the top number, see below) is greater than the denominator (the bottom number, see below).

To define numerator and denominator, a picture paints a thousand words:-

Here are the 5 easy steps to the subtraction of fractions illustrated by an example:-

In this example 2¼ is a mixed number. To convert a mixed number into an improper fraction:-

1) Multiply the denominator of the fraction (in this case 4) by the whole number:-

2 x 4 = 8

2) Add the answer from 1) to the numerator of the fraction (in this case 1):-

8 + 1 = 9

3) Place the answer from 2) over the denominator of the fraction. Therefore 2¼ expressed as an improper fraction = 9/4

2¼ = 9/4

Remember our example is 2¼ - 3/7 = 9/4 - 3/7.

With simple examples a common denominator may just jump at you. But we need a straightforward method that will work with all fractions. All we have to do is multiply the 2 denominators

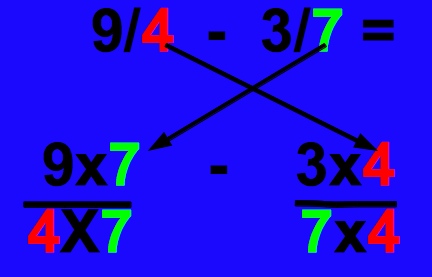
So 9/4 - 3/7 Common denominator = 4 x 7 = 28

Use same amount you multiplied the denominators to multiply the numerators:-

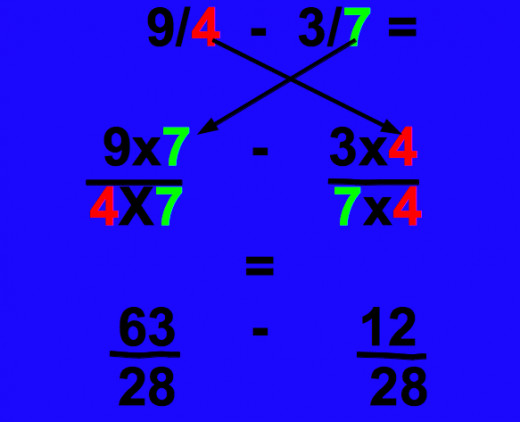
2¼ - 3/7 = 9/4 - 3/7 =

(9 x 7)/(4 x 7) - (3 x 4)/(7 x 4) =

63/28 - 12/28

You need to understand this step, which, is the most challenging step. This is best achieved by just practice. The illustration here will help you understand the logic.

Now the fractions are compatible because the denominators are the same, we can simply subtract one numerator from the other:

First a reminder of the first 3 steps:-

1) Convert mixed numbers to improper fractions:

2¼ - 3/7 = 9/4 - 3/7

2) Find a common denominator:

9/4 - 3/7 Common denominator = 4 x 7 = 28

3) Convert the numerators

9/4 - 3/7 = 63/28 - 12/28

Brings us to:

Step 4, subtract the numerators:-

63/28 - 12/28 = 51/28

This final step is just tidying up your answer. Although the answer from step 4 is technically correct, you may need to tidy up your final answer in order to satisfy your teacher, or much more importantly, your exam board!

51/28 = 1 23/28

Final Answer:-

This hub defined the key terms;- improper fraction, mixed number, denominator and numerator. It then explained the five steps to subtract one fraction from another fraction:-

1. Convert any mixed numbers to improper fractions.
2. Find the common denominators.
3. Convert the numerators by the same factors you converted the denominators
4. Subtract one numerator from the other
5. Simplify and convert any improper fraction answer to a mixed number