Working with rational functions or expressions
Builds on:
What are "rational functions?"
A rational function is one that is expressed as a fraction - this is usually what "rational" means in mathematics.
They are also referred to as rational expressions.
You can expect to see any types of functions involved: linear, quadratic, cubic... The key to any function being a rational function is the fact that one expression is divided by the other.
Simplifying rational functions
To simplify a rational function, the best general advice I can give is: Factorise everything and cancel down. This should always be your first step!
Tip: Don't forget to keep an eye out for the difference of two squares!
Useful links:
- Notes: Rational expressions
Further notes on working with rational expressions. - Practice: Difference of two squares
More on the difference of two squares, with practice questions.
Example:
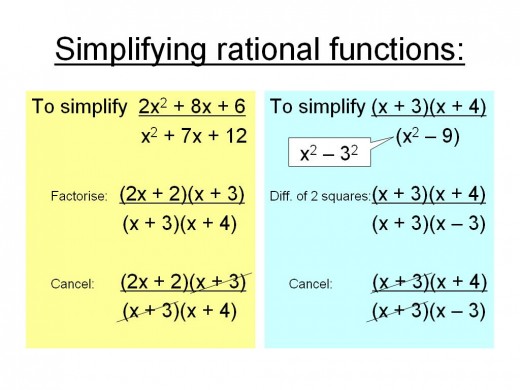
Looking at the first example to the right, if we factorise 2x2 + 8x + 6 we get (2x + 2)(x +3), doing the same to x2 + 7x + 12 gives (x + 3)(x + 4). If we divide these two rewritten expressions, we can see that common factors cancel out - we can remove (x + 3) from the numerator and from the denominator.
This would leave (2x + 2) over (x + 4) as our simplifed rational function.
Example using difference of two squares:
The second example looks harder, until you realise that (x2 - 9) can be factorised using the difference of two squares method; as it's (x2 - 32) we can rewrite it as (x + 3)(x - 3).
After that, simply cancel down as before to leave (x + 4) over (x - 3).