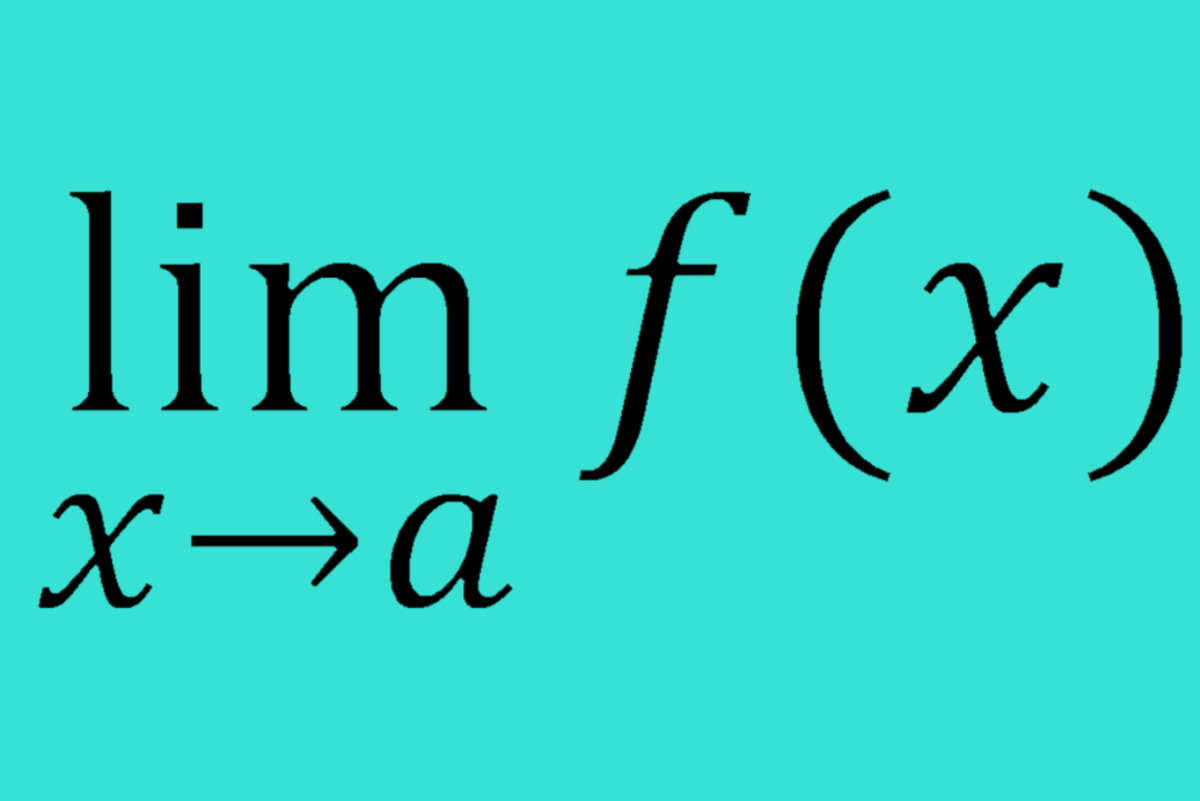The Derivative of a Logarithmic Function and Logarithmic Differentiation:

1. Paragraphs 6, 7, 8 and 9 addresses God, nut, bolts, tires, sinking ships, and windows.

WHAT IS A DERIVATIVE?

2. It tells you how fast something is changing in an instant of time. It can be applied to a myriad of things including velocity and acceleration as with rockets, particles, waves; economics( profit, costs, income); electronics( fields, potential energies, forces), and chemistry( reaction rates, heat exchange, explosion rates). It peers deep into a point of a graph and will tell you the slope( its rate of change) of the graph at that point. Lots of information about things can be found by differentiating functions that describe those things. For example, by differentiating the velocity vector of an electron in its classical Bohr orbit you can determine the centripetal acceleration that electron will experience while in orbit. It is 9 X 10^²² meters per second^², which is 9 billion trillion( 9.174 X 10^21) g-forces. If a feather experienced that many g-forces it would weigh 5,734 trillion tons, and a grain of rice would weigh 158 trillion tons( the weight of 433 million Empire State Buildings). Stable electron orbits is another testimony of God's omniscience in His design of the universe.

WORK FROM L1 THROUGH L6 EXPLAINED:

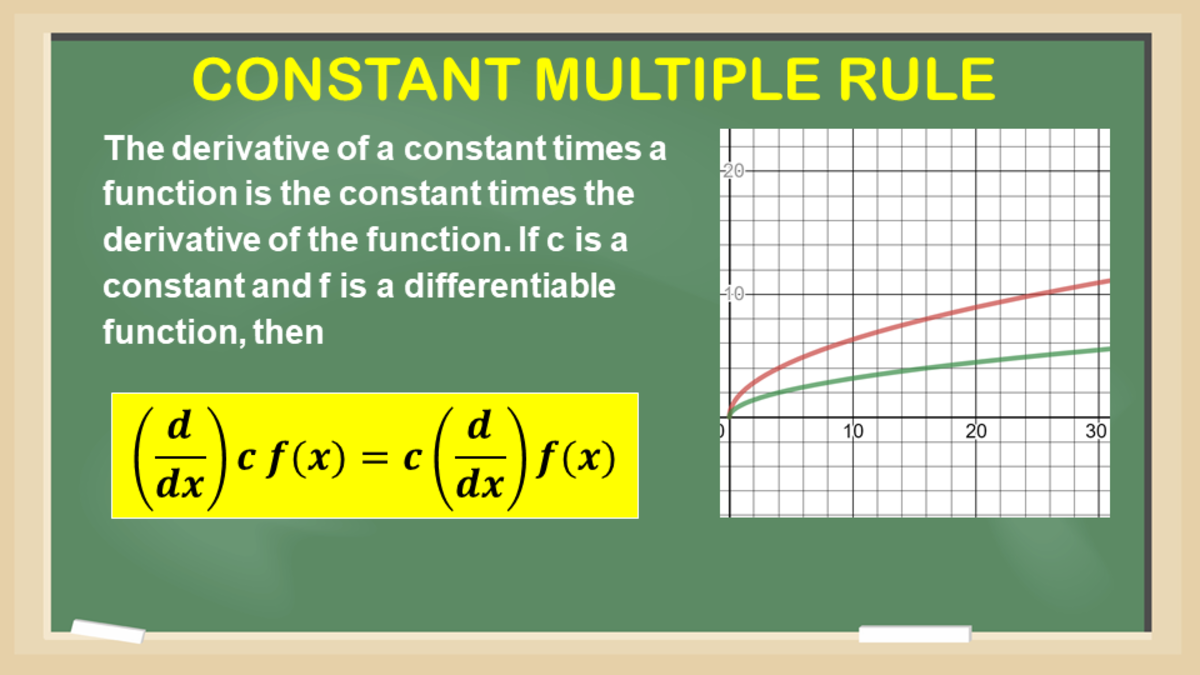
3. To reduce redundancy, refer to hub#12.12 for more information on logarithmic functions. L1 gives the logarithmic function along with the notation for finding its derivative. L2 gives the definition of a derivative. The denominator represents an extremely small change in X, and the numerator represents the corresponding change in Y; therefore, it is rise over run or the slope of a line. At L3 the functions are plugged in, and L4 uses the laws of logarithms to combine the 2 terms in the numerator into one term. I got this derivation from "Calculus and Analytic Geometry, 4th ed." by Douglas F. Riddle, and I think it is brilliant. The denominator is brought to the front as a factor at the end of L4, and L5 multiplies it by 1, X / X. The commutative law of multiplication is used to switch the X and h in the first 2 factors of L6, and the argument( see hub#12.12 ) of the log function is changed with basic laws of arithmetic.

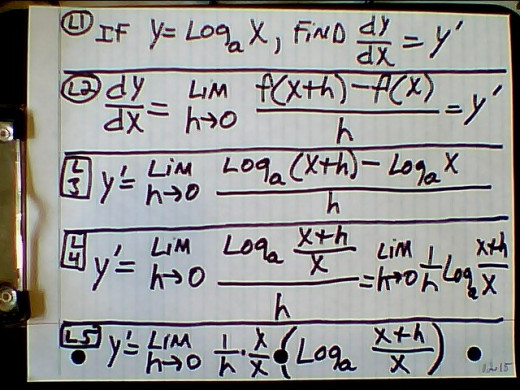
L7 THROUGH L10 EXPLAINED:

4. At L7 the laws of logs tells us we can take the 2nd factor( X / h) of L6 and put it as an exponent of the log's argument. Both factors, the 1 / X and X / h, could go as the exponent, but we only want the 2nd one. This is often done in math--doing a specific manipulation to get a desired result. The argument of the log function at L7 or L10 may look familiar. It is the argument for the limit function that defines e, the base of the natural log function, which is at L1 and L13 of hub#12.14( linked). The exponent goes toward infinity in both cases and the summand goes toward zero in both cases. This fact--e being the argument for the log function--is very important as you will soon see. L8 is using substitution, a handy mathematical technique, to show, via L8 and L9, that L7 and L10 are the same function.

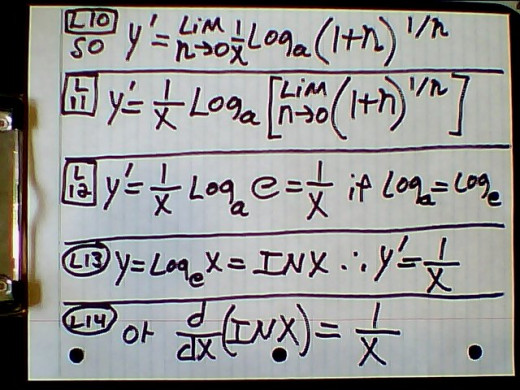
L11 THROUGH L14 EXPLAINED:

5. L11 is the most important step. The laws of limits allows us to put the limit function inside the log function making it part of the argument of the log function. Well, this is the definition of e, as hub#12.14 explains. So at L11 we can put e for the log's argument rather than the limit function. If log_a is to the base e then log_a e = In e, which equals 1 at L12, and 1 / X times 1 is 1 / X. This is our answer at L12. L13 and L14 just show two ways of saying the natural log function and two ways of writing the derivative function.

DERIVATIVES OF LOGARITHMS OF OTHER BASES:

ED1. The derivative of the common logarithm( base 10) is at E1. E2 through E7 shows how this is done. Remember the definition of a logarithm. At E3 we see how it is defined. Log₇X = u means that 7 is raised to what power,u, so that is equals X. If X = 49 then we know that log₇ 49 = 2 because 7 squared equals 49. L12 gives the formula for finding the derivative of a log to any base. All we have to do is evaluate the logarithmic function and then multiply it by 1 over x, and we then have the derivative. To find the value of Log₇e we write the log function in terms of its definition at E3, and then solve for u at E4. X = e in this case; therefore, we can evaluate u at E4 and E5 and find its answer is 0.513898. So 7 raised to this answer will give us e. E7 is just giving another example of this with a log to the base 3.

LOGARITHMIC DIFFERENTIATION:

ED2. Some functions can be very difficult--or at least cumbersome and tedious--to differentiate if not for a remarkable mathematical tool called logarithmic differentiation( LD). I thought L15 would be a good candidate for LD, and an effective representation of how LD works. Frankly, I don't know how L15 can be differentiated without LD.

FOLLOW THE RULES:

ED3. The first thing to do with a problem like this is to follow the rules. Work from the top down with an exponent like this unless parentheses tell you differently. L16 gives an example of what not to do. The exponent for the base 3 is three raised to the x, which is raised to the third power. As we go through the procedure it will become clear how to handle this exponent. L17 is just showing three different symbolic ways of saying the same thing.

TAKE THE LOGARITHM( TWICE) ON BOTH SIDES OF THE EQUATION:

ED4. The exponent is the challenging part of this problem. Even if it was just 3^x we would still use LD to differentiate it. LD begins by taking the logarithm of both sides of the equation. This is done at L18. Notice on the right side that our base is three, and its entire exponent is 3^x^3. Since this is the exponent for its base 3, the rules of logarithms( logs) tell us we can put the exponent in front of the log function, with argument 3( the base). Then the commutative property of multiplication is used to put "IN(3)" in front of the exponent at L18 to remind us that IN(3) is a constant, not a variable. Notice at L18 that 3^x^3 is 3 with its own exponent; therefore, we want to take the log of both sides again so we can get rid of it as an exponent and put it as a factor just as we did for 3^3^x^3 going from L17 to L18. Executing this second log was done at L19, and it is now ready to differentiate. Remember when taking the log of a product, you add the logs of its factors, which was done at L19.

L19 CONTINUED AND L20 EXPLAINED:

ED5. At L19 we can think of In(y) as u; therefore, In(In(y)) = In(u). This differentiated will give 1/u, but the CHAIN RULE must be used; therefore, since u = In(y) then u' = du/dy = 1/y, which was done at L20, but we are not done with the left side of L20. y is a function of x; therefore, we use the chain rule once more to get dy/dx, which finishes the left side of the equation at L20.

ED6. Now for the right side of L20: As fancy as the first term, In(In(3)), looks, it is still a constant; therefore, its derivative is zero; since the rate of change of a constant is zero. I wonder if this is why it is called a constant, what do you think? The second term is just In(3)3x^2 because In(3) is just a constant. The PRODUCT RULE is not used here for that reason.

L21 THROUGH L24 EXPLAINED:

ED7. At L21 we isolate dy/dx by multiplying both sides of the equation at L20 by yIn(y). Executing the commutative property our final answer is at L22. I got this problem out of my textbook, but it was an even numbered problem; therefore, I had no answer for it. My Ti-89 calculator gave me the 2nd line of L22 as an answer. They don't look very much alike, do they? We can plug in a number to see if we get the same answer as what was done at L27 and L28, and as can be seen we do have the same answer, which represents the rate of change( tangent slope) at the curve at x = 1.

ED8. However, I would like to know for sure they are the same answer by algebraic confirmation. First we equate my answer to Ti-89's answer, and right off the bat we can divide both sides of the equation by (3x^2)(In(3) to get rid of them, and we are left with L23. We use the laws of logs to go from L23 to L24. Take a look at L29, and it will give more detail on what was done to go from L23 to L24. We go back to L15 and look at the definition of y, and see that y = 3^3^x^3; therefore, we can divide this common factor out and are left with the 1st line of L25.

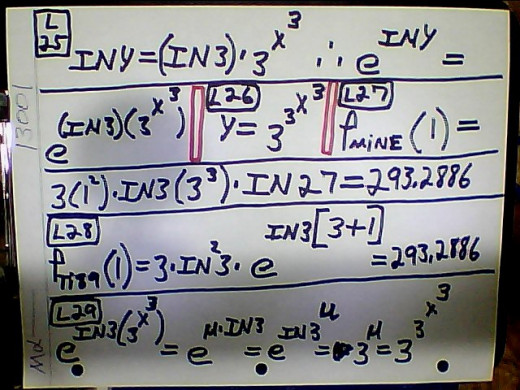
L25 THROUGH L29 EXPLAINED:

ED9. Next at L25 we exponentiate both sides of the equation with the base e. But using the laws of logarithms again we get L26 proving my answer and the Ti-89's answer are the same--and I'm sticking to that story! L27 through L29 have already been explained in the text.

WHO ORDERED THE NUTS AND BOLTS?

6. This is a continuation of paragraph 8 of hub#12.14. As I said in that paragraph( 8), I've used the tornado argument many times; however, it is an enormous understatement. Using that analogy, as it is usually done, is like poking someone with a bazooka rather than actually using the bazooka. We want to calculate the chances of a tornado going through a junkyard and assembling from the parts a fully working Boeing 747 jet aircraft. The chances are impossible--it is not ever going to happen. But this is only half the story. In order to make the nuts and bolts, the minerals had to be mined, transported, and processed. The amount of machinery, organized effort, and man-hours that this required is enormous. Then after the minerals are prepared they can be forged into nuts and bolts of the correct dimensions, the correct thread size, the correct strength, etc. Same goes for the copper wire, all the parts of the instruments, and everything else as the glass windows fitting exactly in the right holes with the correct dimensions. The rubber for the wheels had to be acquired, transported, processed and made to fit. That is one busy tornado!

GOD ORDERED THE NUTS AND BOLTS, WIRE AND TIRES AND WINDOWS, AND . . .

7. Chuck Smith( if memory serves me correctly) told a joke many years ago. It goes something like this: An atheist says to God, Well, we can make life in a test tube. It is really no big deal. God says, OK, do it. So the atheist stoops down to get some dirt, and God says, Oh no. No way. You get your own dirt. You see, the atheists and evolutionists want everything given to them. All the finely tuned forces, the chemical and thermodynamic laws, the amino acids, cell membranes, the DNA, RNA, the stored information, the chemical elements, laws of physics, sub-atomic particles, the precisely adjusted constants of nature( creation); the earth, air, water and gravity, and don't forget the sun. Better throw in the biosphere, because no life will continue without it. They want it all. What is humorous about this is even after they get it all with no argument from Christians, they still cannot build a viable theory of how life, and lifeforms, came into existence without God. With all these tools and parts to play with, they still cannot come up with a theory--that breaks the bounds of laughable--that would explain how Humpty Dumpty was put together by random processes.

A SINKING SHIP CANNOT SAVE LIVES:

8. When the Titanic began to sink, distress call were put out for ships that were still floating. If we were to employ a metaphor to compare the argumentation upon which Darwinism is based to a ship, then we cannot in all honesty use the Titanic as that ship. Perhaps a poorly built raft with rotting twine loosely tied together would be more appropriate. Not that it ever did float, or could float, some people have it in there minds that is does float; however, even in fantasy land where reality is not acknowledged, the raft that could never float in the first place is sinking now. Science is discovering more complexity to explain, not less. Abandon ship all unbelievers before you sink into the abyss and die with the raft that could never keep you afloat in the first place. Get on the ark that can float and save--God's Word. The evidences that He is the Creator are irrefutable and undeniable( Romans 1:20).

SAVING FAITH IS DISCIPLINE:

9. Once one makes a decision to follow Christ, then he must follow Christ--do what He says. This requires discipline. North Wind wrote a hub concerning disobedience and discipline( link to it). In that hub she associates the disobedience of children to authority, but we can all apply it to ourselves and God.